Advertisements
Advertisements
Question
Is it possible to draw a triangle with sides of length 2 cm, 3 cm and 7 cm?
Solution
Given lengths of sides are 2cm, 3cm and 7cm we have to check whether it is possible to draw a triangle with ten the given lengths of sides
We know that,
A triangle can be drawn only when the sum of any two sides is greater than the third side.
So, let’s check the rule
2+3>7 or 2+3<7
2+7>3
and 3+7>2
Here, 2+3>7 So, the triangle does not exit.
APPEARS IN
RELATED QUESTIONS
Prove that the medians of an equilateral triangle are equal.
Find the measure of each exterior angle of an equilateral triangle.
In Fig. 10.131, prove that: (i) CD + DA + AB + BC > 2AC (ii) CD + DA + AB > BC
In a ΔABC, if ∠A = 60°, ∠B = 80° and the bisectors of ∠B and ∠C meet at O, then ∠BOC =
In the given figure, if BP || CQ and AC = BC, then the measure of x is
The angles of a right angled triangle are
Which of the following correctly describes the given triangle?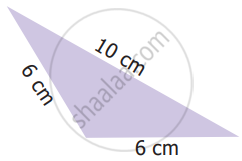
In ∆ABC, BC = AB and ∠B = 80°. Then ∠A is equal to ______.
M is a point on side BC of a triangle ABC such that AM is the bisector of ∠BAC. Is it true to say that perimeter of the triangle is greater than 2 AM? Give reason for your answer.
Is it possible to construct a triangle with lengths of its sides as 9 cm, 7 cm and 17 cm? Give reason for your answer.
