Advertisements
Advertisements
Question
If the angles A, B and C of ΔABC satisfy the relation B − A = C − B, then find the measure of ∠B.
Solution
In the given ΔABC,
∠A, ∠B and ∠C satisfy the relation B - A = C - B
We need to fine the measure of ∠B.
As,
B - A = C - B
B + B = C + A
2B = C + A
2B - A = C ........(1)
Now, using the angle sum property of the triangle, we get,
A + B + C = 180°
2B - A + A +B = 180° (Using 1)
3B = 180°
`B = (180°) / 3`
= 60°
Therefore, ∠B = 60°
APPEARS IN
RELATED QUESTIONS
In a ΔABC, if AB = AC and ∠B = 70°, find ∠A.
The vertical angle of an isosceles triangle is 100°. Find its base angles.
BD and CE are bisectors of ∠B and ∠C of an isosceles ΔABC with AB = AC. Prove that BD = CE.
Prove that each angle of an equilateral triangle is 60°.
ABC is a triangle in which BE and CF are, respectively, the perpendiculars to the sides AC and AB. If BE = CF, prove that ΔABC is isosceles
In the given figure, x + y =

Which of the following correctly describes the given triangle?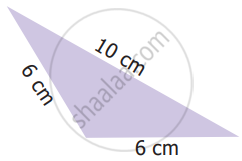
AD is a median of the triangle ABC. Is it true that AB + BC + CA > 2AD? Give reason for your answer.
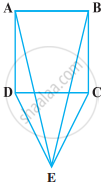
CDE is an equilateral triangle formed on a side CD of a square ABCD (Figure). Show that ∆ADE ≅ ∆BCE.
ABC is an isosceles triangle with AB = AC and D is a point on BC such that AD ⊥ BC (Figure). To prove that ∠BAD = ∠CAD, a student proceeded as follows:

In ∆ABD and ∆ACD,
AB = AC (Given)
∠B = ∠C (Because AB = AC)
and ∠ADB = ∠ADC
Therefore, ∆ABD ≅ ∆ACD (AAS)
So, ∠BAD = ∠CAD (CPCT)
What is the defect in the above arguments?
[Hint: Recall how ∠B = ∠C is proved when AB = AC].
