Advertisements
Advertisements
Question
In the given figure, x + y =

Options
270
230
210
190°
Solution
In the given figure, we need to find x+ y
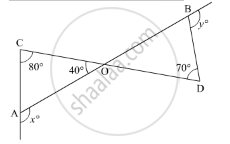
Here, AB and CD are straight lines intersecting at point O, so using the property, “vertically opposite angles are equal”, we get,
∠BOD = ∠AOC
∠BOD = 40°
Further, applying the property, “an exterior angle of a triangle is equal to the sum of the two opposite interior angles”, in ΔAOC, we get,
x° = ∠ACO + ∠AOC
x° = 80° + 40°
x° = 120°
Similarly, in ΔBOD
y° = ∠BOD + ∠BDO
y° = 40° + 70°
y° = 110°
Thus,
x° + y° = 120° + 110°
x° + y° = 230°
APPEARS IN
RELATED QUESTIONS
In an isosceles triangle ABC, with AB = AC, the bisectors of ∠B and ∠C intersect each other at O. Join A to O. Show that:
- OB = OC
- AO bisects ∠A
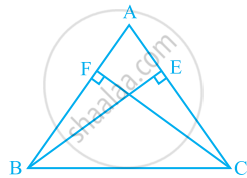
ABC is an isosceles triangle in which altitudes BE and CF are drawn to equal sides AC and AB respectively (see the given figure). Show that these altitudes are equal.
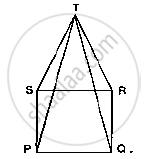
In Fig. 10.23, PQRS is a square and SRT is an equilateral triangle. Prove that
(i) PT = QT (ii) ∠TQR = 15°
P is a point on the bisector of an angle ∠ABC. If the line through P parallel to AB meets BC at Q, prove that triangle BPQ is isosceles.
Which of the following statements are true (T) and which are false (F):
The measure of each angle of an equilateral triangle is 60°
In a ΔABC, if ∠B = ∠C = 45°, which is the longest side?
Which of the following statements are true (T) and which are false (F)?
Of all the line segments that can be drawn from a point to a line not containing it, the perpendicular line segment is the shortest one.
Fill in the blank to make the following statement true.
The sum of three altitudes of a triangle is ..... than its perimeter.
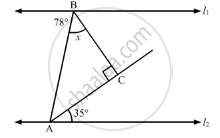
In the given figure, for which value of x is l1 || l2?
ABC is an isosceles triangle with AB = AC and D is a point on BC such that AD ⊥ BC (Figure). To prove that ∠BAD = ∠CAD, a student proceeded as follows:

In ∆ABD and ∆ACD,
AB = AC (Given)
∠B = ∠C (Because AB = AC)
and ∠ADB = ∠ADC
Therefore, ∆ABD ≅ ∆ACD (AAS)
So, ∠BAD = ∠CAD (CPCT)
What is the defect in the above arguments?
[Hint: Recall how ∠B = ∠C is proved when AB = AC].
