Advertisements
Advertisements
Question
ABC is an isosceles triangle in which altitudes BE and CF are drawn to equal sides AC and AB respectively (see the given figure). Show that these altitudes are equal.
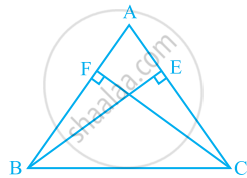
Solution
△ABC is an isosceles triangle.
∴ AB = AC
∠ACB = ∠ABC ...[Angles opposite to equal sides of a △ are equal]
∠BCE = ∠CBF
Now, in △BEC and △CFB
∠BCE = ∠CBF ...[Proved above]
∠BEC = ∠CFB ...[Each 90°]
BC = CB ...[Common]
∴ △BEC ≅ △CFB ...[By AAS congruence]
So, BE = CF ...[By Corresponding parts of congruent triangles]
APPEARS IN
RELATED QUESTIONS
Show that the angles of an equilateral triangle are 60° each.
Two lines AB and CD intersect at O such that BC is equal and parallel to AD. Prove that the lines AB and CD bisect at O.
BD and CE are bisectors of ∠B and ∠C of an isosceles ΔABC with AB = AC. Prove that BD = CE.
O is any point in the interior of ΔABC. Prove that
(i) AB + AC > OB + OC
(ii) AB + BC + CA > OA + QB + OC
(iii) OA + OB + OC >` 1/2`(AB + BC + CA)
Which of the following statements are true (T) and which are false (F)?
Sum of the three sides of a triangle is less than the sum of its three altitudes.
In the given figure, x + y =

In the given figure, if BP || CQ and AC = BC, then the measure of x is
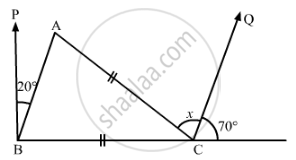
If the bisectors of the acute angles of a right triangle meet at O, then the angle at Obetween the two bisectors is
In the given figure, if l1 || l2, the value of x is

In ∆PQR, ∠P = 70° and ∠R = 30°. Which side of this triangle is the longest? Give reason for your answer.
