Advertisements
Advertisements
प्रश्न
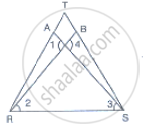
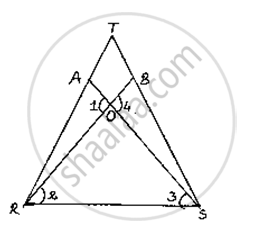
In Fig. 10.40, it is given that RT = TS, ∠1 = 2∠2 and ∠4 = 2∠3. Prove that ΔRBT ≅ ΔSAT
उत्तर
In the figure given that
RT = TS ……..(1)
∠1 = 2∠2 ……..(2)
And∠4 = `2sqrt` ................(3)
And given to prove ΔRBT≅ ΔSAT
Let the point of intersection of RB and SA be denoted by O Since RB and SA intersect at O.
∴∠AOR = ∠BOS [Vertically opposite angles]
⇒∠1 = ∠4
⇒ 2∠2 = 2∠3 [From (2) and (3)
Þ
Þ ∠2 = ∠3 ……..(4)
Now we have RT = TS ∠∠TRS
∴ΔTRS is an isosceles triangle
∴∠TRS = ∠TSR ……..(5) [Angles opposite to equal sides are equal]
But we have
∠TRS = ∠TRB + ∠2 ………(6)
And ∠TSR = ∠TSA + ∠3 ……….(7)
Putting (6) and (7) in (5) we get
∠TRB +∠2 = ∠TSA + ∠B
⇒∠ TRB =∠TSA [∵ From (4)]
Now considerΔRBT and ΔSAT
RT = ST [From (1)]
∠TRB = ∠TSA [From (4)]
∠RTB =∠ STA [Common angle]
From ASA criterion of congruence, we have ΔRBT ≅ΔSAT
APPEARS IN
संबंधित प्रश्न
P is a point on the bisector of an angle ∠ABC. If the line through P parallel to AB meets BC at Q, prove that triangle BPQ is isosceles.
Prove that each angle of an equilateral triangle is 60°.
ABC is a triangle in which ∠B = 2 ∠C. D is a point on BC such that AD bisects ∠BAC and AB = CD.
Prove that ∠BAC = 72°.
Which of the following statements are true (T) and which are false (F):
If the bisector of the vertical angle of a triangle bisects the base, then the triangle may be isosceles.
In ΔABC, if ∠A = 40° and ∠B = 60°. Determine the longest and shortest sides of the triangle.
Is it possible to draw a triangle with sides of length 2 cm, 3 cm and 7 cm?
If the angles of a triangle are in the ratio 2 : 1 : 3, then find the measure of smallest angle.
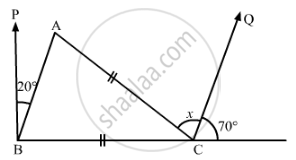
In the given figure, if BP || CQ and AC = BC, then the measure of x is
ABC is an isosceles triangle with AB = AC and D is a point on BC such that AD ⊥ BC (Figure). To prove that ∠BAD = ∠CAD, a student proceeded as follows:

In ∆ABD and ∆ACD,
AB = AC (Given)
∠B = ∠C (Because AB = AC)
and ∠ADB = ∠ADC
Therefore, ∆ABD ≅ ∆ACD (AAS)
So, ∠BAD = ∠CAD (CPCT)
What is the defect in the above arguments?
[Hint: Recall how ∠B = ∠C is proved when AB = AC].
Show that in a quadrilateral ABCD, AB + BC + CD + DA < 2(BD + AC)
