Advertisements
Advertisements
Question
ABC is a right angled triangle in which ∠A = 90° and AB = AC. Find ∠B and ∠C.
Solution
Given that ABC is a right angled triangle such that ∠A = 90° and AB = AC Since,
AB = AC ⇒ ΔABC is also isosceles
∴ We can say that ΔABC is right angled isosceles triangle
⇒ ∠C=∠B and ∠A=90° ................(1)
Now, we have
Sum of angled in a triangle =180°
⇒ ∠A+∠B+∠C=180°
⇒ 90°+∠B+∠B=180° [∵ From (1)]
⇒ 2∠B=180°-90°
⇒`∠B=(90°)/2=45°`
∴ ∠B=∠C=45°

APPEARS IN
RELATED QUESTIONS
Show that the angles of an equilateral triangle are 60° each.
Fill the blank in the following so that the following statement is true.
If altitudes CE and BF of a triangle ABC are equal, then AB = ....
Fill in the blank to make the following statement true.
In a right triangle the hypotenuse is the .... side.
In the given figure, the sides BC, CA and AB of a Δ ABC have been produced to D, E and F respectively. If ∠ACD = 105° and ∠EAF = 45°, find all the angles of the Δ ABC.
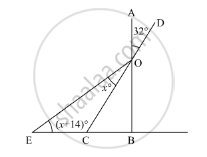
In the given figure, if AB ⊥ BC. then x =
In ∆PQR, if ∠R > ∠Q, then ______.
In triangles ABC and PQR, AB = AC, ∠C = ∠P and ∠B = ∠Q. The two triangles are ______.
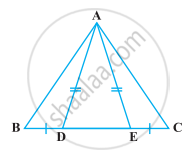
In the following figure, D and E are points on side BC of a ∆ABC such that BD = CE and AD = AE. Show that ∆ABD ≅ ∆ACE.
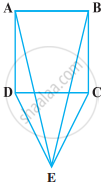
CDE is an equilateral triangle formed on a side CD of a square ABCD (Figure). Show that ∆ADE ≅ ∆BCE.
ABC is an isosceles triangle with AB = AC and D is a point on BC such that AD ⊥ BC (Figure). To prove that ∠BAD = ∠CAD, a student proceeded as follows:

In ∆ABD and ∆ACD,
AB = AC (Given)
∠B = ∠C (Because AB = AC)
and ∠ADB = ∠ADC
Therefore, ∆ABD ≅ ∆ACD (AAS)
So, ∠BAD = ∠CAD (CPCT)
What is the defect in the above arguments?
[Hint: Recall how ∠B = ∠C is proved when AB = AC].
