Advertisements
Advertisements
Question
In two triangles ABC and ADC, if AB = AD and BC = CD. Are they congruent?
Solution
The given information and corresponding figure is given below
AB = AD
BC = CD

From the figure, we have
AB = AD (given)
CB = CD (given)
And,
AC = AC (common sides)
Hence, triangles ABC and ADC are congruent to each other.
APPEARS IN
RELATED QUESTIONS
ABCD is a quadrilateral in which AD = BC and ∠DAB = ∠CBA (See the given figure). Prove that
- ΔABD ≅ ΔBAC
- BD = AC
- ∠ABD = ∠BAC.

AB is a line segment and P is its mid-point. D and E are points on the same side of AB such that ∠BAD = ∠ABE and ∠EPA = ∠DPB (See the given figure). Show that
- ΔDAP ≅ ΔEBP
- AD = BE
You want to show that ΔART ≅ ΔPEN,
If it is given that AT = PN and you are to use ASA criterion, you need to have
1) ?
2) ?

In Δ ABC, ∠B = 35°, ∠C = 65° and the bisector of ∠BAC meets BC in P. Arrange AP, BP and CP in descending order.
In triangles ABC and CDE, if AC = CE, BC = CD, ∠A = 60°, ∠C = 30° and ∠D = 90°. Are two triangles congruent?
The following figure shows a circle with center O.
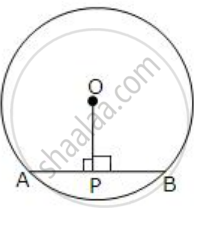
If OP is perpendicular to AB, prove that AP = BP.
In the following figure, BL = CM.

Prove that AD is a median of triangle ABC.
In the following diagram, ABCD is a square and APB is an equilateral triangle.
(i) Prove that: ΔAPD ≅ ΔBPC
(ii) Find the angles of ΔDPC.
A point O is taken inside a rhombus ABCD such that its distance from the vertices B and D are equal. Show that AOC is a straight line.
ABC is a right triangle with AB = AC. Bisector of ∠A meets BC at D. Prove that BC = 2AD.
