Advertisements
Advertisements
Question
Find the radius of gyration of circular ring of radius r about a line perpendicular to the plane of the ring and passing through one of its particles.
Solution
Moment of inertia of the ring about a point on the rim of the ring and the axis perpendicular to the plane of the ring = mR2 + mR2 = 2mR2 (from parallel axis theorem)
We know that
\[m K^2 = 2m R^2 \]
K = Radius of the gyration
\[ \Rightarrow K = \sqrt{2 R^2} = \sqrt{2}R\]
APPEARS IN
RELATED QUESTIONS
State the theorem of perpendicular axes about moment of inertia.
State an expression for the moment of intertia of a solid uniform disc, rotating about an axis passing through its centre, perpendicular to its plane. Hence derive an expression for the moment of inertia and radius of gyration:
i. about a tangent in the plane of the disc, and
ii. about a tangent perpendicular to the plane of the disc.
State Brewster's law.
Prove the theorem of perpendicular axes.
(Hint: Square of the distance of a point (x, y) in the x–y plane from an axis through the origin perpendicular to the plane is x2 + y2).
Prove the theorem of parallel axes.
(Hint: If the centre of mass is chosen to be the origin `summ_ir_i = 0`
Answer in brief:
State the conditions under which the theorems of parallel axes and perpendicular axes are applicable. State the respective mathematical expressions.
A string of length ℓ fixed at one end carries a mass m at the other. The string makes 2/π revolutions/sec around the vertical axis through the fixed end. The tension in the string is ______.
A solid cylinder of radius r and mass M rolls down an inclined plane of height h. When it reaches the bottom of the plane, then its rotational kinetic energy is ____________.
(g = acceleration due to gravity)
When a 12000 joule of work is done on a flywheel, its frequency of rotation increases from 10 Hz to 20 Hz. The moment of inertia of flywheel about its axis of rotation is ______. (π2 = 10)
A circular disc 'X' of radius 'R' made from iron plate of thickness 't' has moment of inertia 'Ix' about an axis passing through the centre of disc and perpendicular to its plane. Another disc 'Y' of radius '3R' made from an iron plate of thickness `("t"/3)` has moment of inertia 'Iy' about the s same as that of disc X. The relation between Ix and ly is ______.
Two bodies have their moments of inertia I and 2I respectively about their axes of rotation. If their kinetic energies of rotation are equal, their angular momenta will be in the ratio ______.
A wheel of moment of inertia 2 kg m2 is rotating at a speed of 25 rad/s. Due to friction on the axis, it comes to rest in 10 minutes. Total work done by friction is ______.
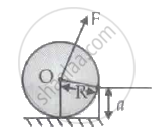
A uniform cylinder of mass M and radius R is to be pulled over a step of height a (a < R) by applying a force F at its centre 'O' perpendicular to the plane through the axes of the cylinder on the edge of the step (see figure). The minimum value of F required is:
Two particles A and B having equal charges are placed at a distance d apart. A third charged particle placed on the perpendicular bisection of AB at distance x. The third particle experiences maximum force when ______.
A uniform disc of mass 10 kg and radius 60 cm rotates about an axis perpendicular to its plane and passing through its centre at 1200 rpm. Calculate its rotation kinetic energy. [Take π2 = 10]
Prove the theorem of perpendicular axes about the moment of inertia.
