Advertisements
Advertisements
Question
Find the sum of the lengths of the bases of a trapezium whose area is 4.2 m2 and whose height is 280 cm.
Solution
Area of the trapezium = 4.2 m2
Height = 280 cm \[=\frac{280}{100}m = 2.8 m\]
Area of trapezium\[= \frac{1}{2} \times(\text{ Sum of the parallel bases })\times(\text{ Height })\]
\[4.2 =\frac{1}{2}\times(\text{ Sum of the parallel bases })\times2.8\]
\[4 . 2 \times 2 = (\text{ Sum of the parallel bases })\times2.8\]
\[\text{ Sum of the parallel bases }= \frac{8 . 4}{2 . 8} = 3 m\]
APPEARS IN
RELATED QUESTIONS
The area of a trapezium is 34 cm2 and the length of one of the parallel sides is 10 cm and its height is 4 cm. Find the length of the other parallel side.
Find the area, in square metres, of the trapezium whose bases and altitude is as under:
bases = 8 m and 60 dm, altitude = 40 dm
Find the area of trapezium with base 15 cm and height 8 cm, if the side parallel to the given base is 9 cm long.
Find the height of a trapezium, the sum of the lengths of whose bases (parallel sides) is 60 cm and whose area is 600 cm2.
Find the altitude of a trapezium whose area is 65 cm2 and whose bases are 13 cm and 26 cm.
Top surface of a table is trapezium in shape. Find its area if its parallel sides are 1 m and 1.2 m and perpendicular distance between them is 0.8 m.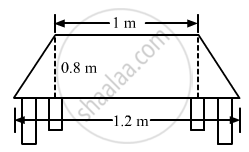
If the area of a trapezium is 28 cm2 and one of its parallel sides is 6 cm, find the other parallel side if its altitude is 4 cm.
The following figure shows the cross-section ABCD of a swimming pool which is a trapezium in shape.
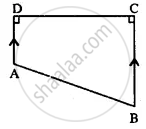
If the width DC, of the swimming pool, is 6.4 m, depth (AD) at the shallow end is 80 cm and depth (BC) at the deepest end is 2.4 m, find its area of the cross-section.
In a trapezium if the sum of the parallel sides is 10 cm and the area is 140 sq.cm, then the height is
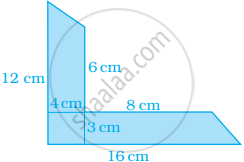
Find the area of the shaded portion in the following figure.