Advertisements
Advertisements
Question
Find the area enclosed by the curve x = 3 cost, y = 2 sint.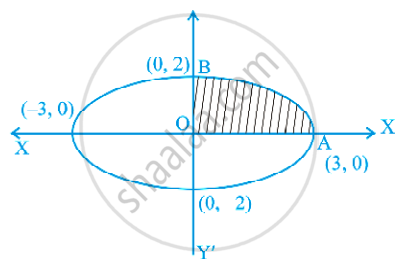
Solution
Eliminating t as follows:
x = 3 cost
y = 2 sint
⇒ `x/3` = cos t
`y/2` = sin t
We obtain `x^2/9 + y^2/4` = 1.
Which is the equation of an ellipse.
From the figure in the question, we get
The required area = `4 int_0^3 2/3 sqrt(9 - x^2) "d"x`
= `8/3 [x/2 sqrt(9 - x^2) + 9/2 sin^-1 x/3]_0^3`
= 6π sq.units
APPEARS IN
RELATED QUESTIONS
Find the area bounded by curves (x – 1)2 + y2 = 1 and x2 + y 2 = 1
Find the area of the region bounded by the curves y = x2 + 2, y = x, x = 0 and x = 3
Using integration finds the area of the region bounded by the triangle whose vertices are (–1, 0), (1, 3) and (3, 2).
Smaller area enclosed by the circle x2 + y2 = 4 and the line x + y = 2 is
A. 2 (π – 2)
B. π – 2
C. 2π – 1
D. 2 (π + 2)
Area lying between the curve y2 = 4x and y = 2x is
A. 2/3
B. 1/3
C. 1/4
D. 3/4
Find the area bounded by curves {(x, y) : y ≥ x2 and y = |x|}.
Choose the correct answer The area of the circle x2 + y2 = 16 exterior to the parabola y2 = 6x is
A. `4/3 (4pi - sqrt3)`
B. `4/3 (4pi + sqrt3)`
C. `4/3 (8pi - sqrt3)`
D.`4/3 (4pi + sqrt3)`
The area enclosed between the curves y = loge (x + e), x = loge \[\left( \frac{1}{y} \right)\] and the x-axis is _______ .
The area between x-axis and curve y = cos x when 0 ≤ x ≤ 2 π is ___________ .
Solve the following :
Find the area of the region lying between the parabolas :
y2 = 4x and x2 = 4y
The area of the region included between the parabolas y2 = 16x and x2 = 16y, is given by ______ sq.units
Find the area enclosed between y = cos x and X-axis between the lines x = `pi/2` and x ≤ `(3pi)/2`
Find the area of the ellipse `x^2/1 + y^2/4` = 1, in first quadrant
Find the area of sector bounded by the circle x2 + y2 = 25, in the first quadrant−
Find the area enclosed between the X-axis and the curve y = sin x for values of x between 0 to 2π
Find the area of the ellipse `x^2/36 + y^2/64` = 1, using integration
Find the area of the region included between y = x2 + 5 and the line y = x + 7
Find the area enclosed between the circle x2 + y2 = 9, along X-axis and the line x = y, lying in the first quadrant
Find the area of the region included between the parabola y = `(3x^2)/4` and the line 3x – 2y + 12 = 0.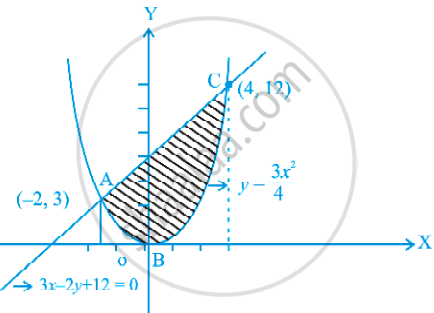
Draw a rough sketch of the curve y = `sqrt(x - 1)` in the interval [1, 5]. Find the area under the curve and between the lines x = 1 and x = 5.
Determine the area under the curve y = `sqrt("a"^2 - x^2)` included between the lines x = 0 and x = a.
Area lying between the curves `y^2 = 4x` and `y = 2x`
The value of a for which the area between the curves y2 = 4ax and x2 = 4ay is 1 sq.unit, is ______.
Using Integration, find the area of triangle whose vertices are (– 1, 1), (0, 5) and (3, 2).
Find the area enclosed between 3y = x2, X-axis and x = 2 to x = 3.
Find the area cut off from the parabola 4y = 3x2 by the line 2y = 3x + 12.
