Advertisements
Advertisements
Question
Find the area of the region bounded by the curves y = x2 + 2, y = x, x = 0 and x = 3
Solution
The area bounded by the curves, y = x2 + 2, y = x, x = 0, and x = 3, is represented by the shaded area OCBAO as
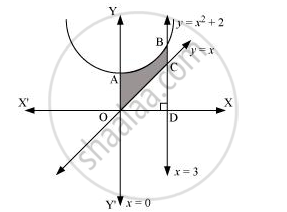
Then, Area OCBAO = Area ODBAO – Area ODCO
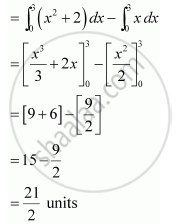
APPEARS IN
RELATED QUESTIONS
Using integration, find the area of the region bounded by the triangle whose vertices are (−1, 2), (1, 5) and (3, 4).
Find the area of the region lying between the parabolas y2 = 4ax and x2 = 4ay.
Find the area bounded by curves (x – 1)2 + y2 = 1 and x2 + y 2 = 1
Smaller area enclosed by the circle x2 + y2 = 4 and the line x + y = 2 is
A. 2 (π – 2)
B. π – 2
C. 2π – 1
D. 2 (π + 2)
Choose the correct answer The area of the circle x2 + y2 = 16 exterior to the parabola y2 = 6x is
A. `4/3 (4pi - sqrt3)`
B. `4/3 (4pi + sqrt3)`
C. `4/3 (8pi - sqrt3)`
D.`4/3 (4pi + sqrt3)`
The area between x-axis and curve y = cos x when 0 ≤ x ≤ 2 π is ___________ .
Area enclosed between the curve y2 (2a − x) = x3 and the line x = 2a above x-axis is ___________ .
Area lying between the curves y2 = 4x and y = 2x is
Solve the following :
Find the area of the region lying between the parabolas :
y2 = 4x and x2 = 4y
The area of the region included between the parabolas y2 = 16x and x2 = 16y, is given by ______ sq.units
The area enclosed between the two parabolas y2 = 20x and y = 2x is ______ sq.units
Find the area of the ellipse `x^2/1 + y^2/4` = 1, in first quadrant
Find the area of sector bounded by the circle x2 + y2 = 25, in the first quadrant−
Find the area enclosed between the X-axis and the curve y = sin x for values of x between 0 to 2π
Find the area of the ellipse `x^2/36 + y^2/64` = 1, using integration
Find the area of the region included between y = x2 + 5 and the line y = x + 7
Find the area enclosed between the circle x2 + y2 = 9, along X-axis and the line x = y, lying in the first quadrant
Find the area of the region included between the parabola y = `(3x^2)/4` and the line 3x – 2y + 12 = 0.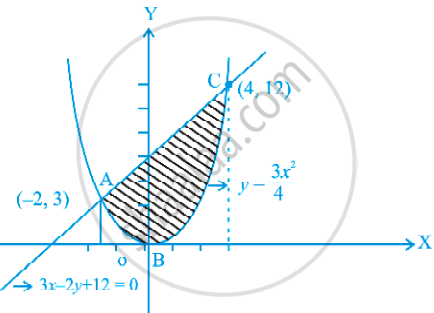
Find the area of the region bounded by the curves x = at2 and y = 2at between the ordinate corresponding to t = 1 and t = 2.
Calcualte the area under the curve y = `2sqrt(x)` included between the lines x = 0 and x = 1
Area lying between the curves `y^2 = 4x` and `y = 2x`
The value of a for which the area between the curves y2 = 4ax and x2 = 4ay is 1 sq.unit, is ______.
Let the area enclosed by the x-axis, and the tangent and normal drawn to the curve 4x3 – 3xy2 + 6x2 – 5xy – 8y2 + 9x + 14 = 0 at the point (–2, 3) be A. Then 8A is equal to ______.
Using Integration, find the area of triangle whose vertices are (– 1, 1), (0, 5) and (3, 2).
