Advertisements
Advertisements
Question
Give a definition of the following term. Are there other terms that need to be defined first? What are they, and how might you define them?
perpendicular lines
Solution
Yes, we need to understand terms like point, line, ray, angle, plane, circle, and quadrilateral before defining the required terms.
Two lines in the same plane, p and q, are considered perpendicular if they make a right angle and are written as p ⊥ q.

APPEARS IN
RELATED QUESTIONS
In the following figure, if AC = BD, then prove that AB = CD.

How many least number of distinct points determine a unique plane?
How many planes can be made to pass through two points?
In ancient India, the shapes of altars used for household rituals were ______.
“For every line l and for every point P not lying on a given line l, there exists a unique line m passing through P and parallel to l ” is known as Playfair’s axiom.
Solve the following question using appropriate Euclid’s axiom:
Two salesmen make equal sales during the month of August. In September, each salesman doubles his sale of the month of August. Compare their sales in September.
Solve the following question using appropriate Euclid’s axiom:
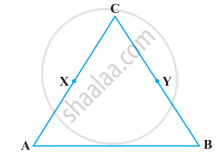
In the following figure, we have X and Y are the mid-points of AC and BC and AX = CY. Show that AC = BC.
In the following figure BM = BN, M is the mid-point of AB and N is the mid-point of BC. Show that AB = BC.

Read the following statement :
An equilateral triangle is a polygon made up of three line segments out of which two line segments are equal to the third one and all its angles are 60° each.
Define the terms used in this definition which you feel necessary. Are there any undefined terms in this? Can you justify that all sides and all angles are equal in a equilateral triangle.
Read the following axioms:
- Things which are equal to the same thing are equal to one another.
- If equals are added to equals, the wholes are equal.
- Things which are double of the same thing are equal to one another.
Check whether the given system of axioms is consistent or inconsistent.
