Advertisements
Advertisements
Question
How many planes can be made to pass through two points?
Solution
Given two distinct points, we can draw many planes passing through them. Therefore, infinite number of planes can be drawn passing through two distinct points or two points can be common to infinite number of planes.
APPEARS IN
RELATED QUESTIONS
Consider two ‘postulates’ given below:-
- Given any two distinct points A and B, there exists a third point C which is in between A and B.
- There exist at least three points that are not on the same line.
Do these postulates contain any undefined terms? Are these postulates consistent? Do they follow from Euclid’s postulates? Explain.
In the following figure, if AC = BD, then prove that AB = CD.

Why is Axiom 5, in the list of Euclid’s axioms, considered a ‘universal truth’? (Note that the question is not about the fifth postulate.)
The number of dimensions, a surface has ______.
Boundaries of solids are ______.
The side faces of a pyramid are ______.
Greek’s emphasised on ______.
Attempts to prove Euclid’s fifth postulate using the other postulates and axioms led to the discovery of several other geometries.
Solve the following question using appropriate Euclid’s axiom:
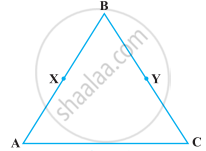
In the following figure, we have BX = `1/2` AB, BY = `1/2` BC and AB = BC. Show that BX = BY.
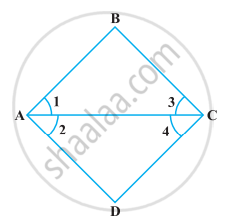
Solve the following question using appropriate Euclid’s axiom:
In the following figure, we have ∠1 = ∠3 and ∠2 = ∠4. Show that ∠A = ∠C.