Advertisements
Advertisements
Question
The cross section of a tunnel perpendicular to its length is a trapezium ABCD as shown in the figure. AM = BN; AB = 4.4 m, CD = 3 m The height of a tunnel is 2.4 m. The tunnel is 5.4 m long. Calculate the cost of painting the internal surface of the tunnel (excluding the floor) at the rate of Rs. 5 per m2.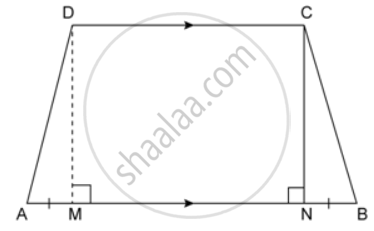
Solution
The internal surface area will consist of faces formed by 1 side as length and other sides as AD, CD and BC.
AM = `(1)/(2)("AB" - "CD")`
= `(1)/(2)(4.4 -3)`
AM = 0.7
In ΔAMD, by Pythagoras theorem,
AD2 = AM2 + DM2
AD2 = (0.7)2 + (2.4)2
AD = 2.5m
AD = BC = 2.5n
Total surface area
= (length x AD) + (length x CD) + (length x BC)
= 5.4(AD + CD + BC)
= 5.4(2.5 + 3 + 2.5)
= 43.2m2
Cost of painting
= 43.2 x 5
= Rs,216
∴ The cost of painting the internal surface is Rs.216.
APPEARS IN
RELATED QUESTIONS
The following figure shows a solid of uniform cross-section. Find the volume of the solid. All measurements are in centimeters.
Assume that all angles in the figures are right angles.
The following figure shows a closed victory-stand whose dimensions are given in cm.
Find the volume and the surface area of the victory stand.
The cross-section of a piece of metal 4 m in length is shown below. Calculate :
(i) The area of the cross-section;
(ii) The volume of the piece of metal in cubic centimeters.
If 1 cubic centimeter of the metal weighs 6.6 g, calculate the weight of the piece of metal to the nearest kg.
The internal dimensions of a rectangular box are 12 cm x `x` cm x 9 cm. If the length of the longest rod that can be placed in this box is 17 cm; find `x`.
Find the length of 22 kg copper wire of diameter 0.8 cm, if the weight of 1 cm3 copper is 4.2 g.
The cross section of a piece of metal 2 m in length is shown. Calculate the area of cross section.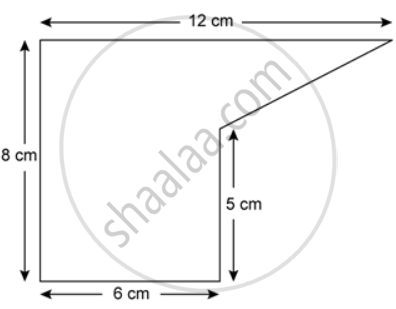
The cross section of a tunnel perpendicular to its length is a trapezium ABCD as shown in the figure. AM = BN; AB = 4.4 m, CD = 3 m The height of a tunnel is 2.4 m. The tunnel is 5.4 m long. Calculate the cost of flooring at the rate of Rs.2. 5 per m2.

ABCDE is the end view of a factory shed which is 50 m long. The roofing of the shed consists of asbestos sheets as shown in the figure. The two ends of the shed are completely closed by brick walls.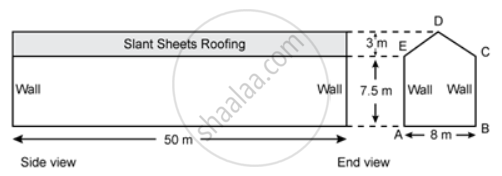
Find the total surface area (including roofing) of the shed.
The cross section of a swimming pool is a trapezium whose shallow and deep ends are 1 m and 3 m respectively. If the length of the pool is 50 m and its width is 1.5 m, calculate the volume of water it holds.
A hose-pipe of cross section area 3 cm2 delivers 1800 liters of water in 10 minutes. Find the speed of water in km/h through the pipe.
