Advertisements
Advertisements
Question
How does the fringe width get affected, if the entire experimental apparatus of Young is immersed in water?
Solution
Fringe width is the distance between two consecutive dark or bright fringes,
so we have fringe width `=(lambdaD)/d`.
If the whole apparatus is immersed in water and refractive index of water is n then,
`v/c =1/n` = Where v is velocity of light in water
`=> n =(vlambda)/(vlambda_omega) lambda =` wavelength of light in air
`=> n =(vlambda)/(lambda_omega) lambda_omega =` wavelength of light in wetar
`lambda_omega = lambda/n v ` = frequency of light in air and water
Hence
`beta_omega =(lambda_omegad)/D =(lambda) =(lamdad)/(nD)`
`beta_omega = 1/n beta`
This shows fringe width will be reduced by the factor of the refractive index of water.
APPEARS IN
RELATED QUESTIONS
In a double-slit experiment the angular width of a fringe is found to be 0.2° on a screen placed 1 m away. The wavelength of light used is 600 nm. What will be the angular width of the fringe if the entire experimental apparatus is immersed in water? Take refractive index of water to be 4/3.
Using analytical method for interference bands, obtain an expression for path difference between two light waves.
A monochromatic light of wavelength 500 nm is incident normally on a single slit of width 0.2 mm to produce a diffraction pattern. Find the angular width of the central maximum obtained on the screen.
Estimate the number of fringes obtained in Young's double slit experiment with fringe width 0.5 mm, which can be accommodated within the region of total angular spread of the central maximum due to single slit.
A Young's double slit experiment is performed with white light.
(a) The central fringe will be white.
(b) There will not be a completely dark fringe.
(c) The fringe next to the central will be red.
(d) The fringe next to the central will be violet.
In a Young's double slit experiment, two narrow vertical slits placed 0.800 mm apart are illuminated by the same source of yellow light of wavelength 589 nm. How far are the adjacent bright bands in the interference pattern observed on a screen 2.00 m away?
In a Young's double slit experiment, the separation between the slits = 2.0 mm, the wavelength of the light = 600 nm and the distance of the screen from the slits = 2.0 m. If the intensity at the centre of the central maximum is 0.20 W m−2, what will be the intensity at a point 0.5 cm away from this centre along the width of the fringes?
Consider the arrangement shown in the figure. By some mechanism, the separation between the slits S3 and S4 can be changed. The intensity is measured at the point P, which is at the common perpendicular bisector of S1S2 and S2S4. When \[z = \frac{D\lambda}{2d},\] the intensity measured at P is I. Find the intensity when z is equal to
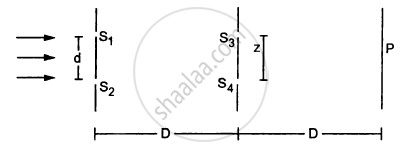
(a) \[\frac{D\lambda}{d}\]
(b) \[\frac{3D\lambda}{2d}\] and
(c) \[\frac{2D\lambda}{d}\]
What should be the path difference between two waves reaching a point for obtaining constructive interference in Young’s Double Slit experiment ?
Young's double slit experiment is made in a liquid. The 10th bright fringe lies in liquid where 6th dark fringe lies in vacuum. The refractive index of the liquid is approximately
Interference fringes are observed on a screen by illuminating two thin slits 1 mm apart with a light source (λ = 632.8 nm). The distance between the screen and the slits is 100 cm. If a bright fringe is observed on a screen at distance of 1.27 mm from the central bright fringe, then the path difference between the waves, which are reaching this point from the slits is close to :
