Advertisements
Advertisements
Question
If 2 and -2 are two zeroes of the polynomial `(x^4 + x^3 – 34x^2 – 4x + 120)`, find all the zeroes of the given polynomial.
Solution
Let f(x) = x^4 + x^3 – 34x^2 – 4x + 120
Since 2 and -2 are the zeroes of f(x), it follows that each one of (x – 2) and (x + 2) is a factor of f(x).
Consequently, (x – 2) (x + 2) = (x2 – 4) is a factor of f(x).
On dividing f(x) by `(x^2 – 4)`, we get:
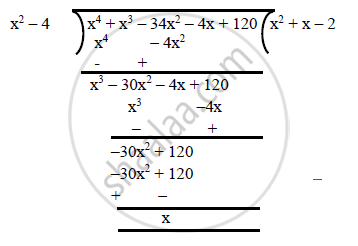
f(x) = 0
⇒` (x2 + x – 30) (x^2 – 4) = 0`
⇒` (x^2 + 6x – 5x – 30) (x – 2) (x + 2)`
⇒ `[x(x + 6) – 5(x + 6)] (x – 2) (x + 2)`
⇒`(x – 5) (x + 6) (x – 2) (x + 2) = 0`
⇒` x = 5 or x = -6 or x = 2 or x = -2`
Hence, all the zeroes are 2, -2, 5 and -6.
APPEARS IN
RELATED QUESTIONS
Find the zeroes of the following quadratic polynomial and verify the relationship between the zeroes and the coefficients:
t2 – 15
If α and β are the zeros of the quadratic polynomial f(x) = 6x2 + x − 2, find the value of `alpha/beta+beta/alpha`.
If 𝛼 and 𝛽 are the zeros of the quadratic polynomial p(x) = 4x2 − 5x −1, find the value of α2β + αβ2.
If 𝛼 and 𝛽 are the zeros of the quadratic polynomial f(x) = x2 − 5x + 4, find the value of `1/alpha+1/beta-2alphabeta`
If α and β are the zeroes of the polynomial f(x) = x2 + px + q, form a polynomial whose zeroes are (α + β)2 and (α − β)2.
If f(x) =` x^4 – 3x^2 + 4x + 5` is divided by g(x)= `x^2 – x + 1`
By actual division, show that x2 – 3 is a factor of` 2x^4 + 3x^3 – 2x^2 – 9x – 12.`
Given that one of the zeroes of the cubic polynomial ax3 + bx2 + cx + d is zero, the product of the other two zeroes is ______.
Find the zeroes of the following polynomials by factorisation method and verify the relations between the zeroes and the coefficients of the polynomials:
`4x^2 + 5sqrt(2)x - 3`
Find the zeroes of the following polynomials by factorisation method and verify the relations between the zeroes and the coefficients of the polynomials:
`y^2 + 3/2 sqrt(5)y - 5`
