Advertisements
Advertisements
प्रश्न
If 2 and -2 are two zeroes of the polynomial `(x^4 + x^3 – 34x^2 – 4x + 120)`, find all the zeroes of the given polynomial.
उत्तर
Let f(x) = x^4 + x^3 – 34x^2 – 4x + 120
Since 2 and -2 are the zeroes of f(x), it follows that each one of (x – 2) and (x + 2) is a factor of f(x).
Consequently, (x – 2) (x + 2) = (x2 – 4) is a factor of f(x).
On dividing f(x) by `(x^2 – 4)`, we get:
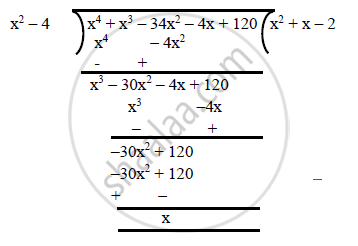
f(x) = 0
⇒` (x2 + x – 30) (x^2 – 4) = 0`
⇒` (x^2 + 6x – 5x – 30) (x – 2) (x + 2)`
⇒ `[x(x + 6) – 5(x + 6)] (x – 2) (x + 2)`
⇒`(x – 5) (x + 6) (x – 2) (x + 2) = 0`
⇒` x = 5 or x = -6 or x = 2 or x = -2`
Hence, all the zeroes are 2, -2, 5 and -6.
APPEARS IN
संबंधित प्रश्न
Find a quadratic polynomial with the given numbers as the sum and product of its zeroes respectively.
`0, sqrt5`
Find all zeroes of the polynomial `(2x^4 - 9x^3 + 5x^2 + 3x - 1)` if two of its zeroes are `(2 + sqrt3)` and `(2 - sqrt3)`
If α and β are the zeros of the quadratic polynomial f(x) = x2 − 1, find a quadratic polynomial whose zeroes are `(2alpha)/beta" and "(2beta)/alpha`
Find the zeroes of the quadratic polynomial f(x) = 4x2 - 4x - 3 and verify the relation between its zeroes and coefficients.
Verify that 3, -2, 1 are the zeros of the cubic polynomial `p(x) = (x^3 – 2x2 – 5x + 6)` and verify the relation between it zeros and coefficients.
On dividing `3x^3 + x^2 + 2x + 5` is divided by a polynomial g(x), the quotient and remainder are (3x – 5) and (9x + 10) respectively. Find g(x).
If 𝛼, 𝛽 are the zeroes of the polynomial f(x) = x2 + x – 2, then `(∝/β-∝/β)`
Given that the zeroes of the cubic polynomial x3 – 6x2 + 3x + 10 are of the form a, a + b, a + 2b for some real numbers a and b, find the values of a and b as well as the zeroes of the given polynomial.
If α, β are zeroes of the quadratic polynomial x2 – 5x + 6, form another quadratic polynomial whose zeroes are `1/α, 1/β`.
If α, β are zeroes of quadratic polynomial 5x2 + 5x + 1, find the value of α–1 + β–1.
