Advertisements
Advertisements
Question
If A(6, 1), B(8, 2), C(9, 4) and D(7, 3) are the vertices of
Solution:
Slope of line =
∴ Slope of line AB =
∴ Slope of line BC =
∴ Slope of line CD =
∴ Slope of line DA =
∴ Slope of line AB =
∴ line AB || line CD
∴ Slope of line BC =
∴ line BC || line DA
Both the pairs of opposite sides of the quadrilateral are parallel.
∴
Solution
Slope of line =
∴ Slope of line AB =
∴ Slope of line BC =
∴ Slope of line CD =
∴ Slope of line DA =
∴ Slope of line AB = Slope of line CD ......[From (i) and (iii)]
∴ line AB || line CD
∴ Slope of line BC = Slope of line DA ......[From (ii) and (iv)]
∴ line BC || line DA
Both the pairs of opposite sides of the quadrilateral are parallel.
∴
APPEARS IN
RELATED QUESTIONS
Find the slope of the line with inclination 30° .
The slope of a line joining P(6, k) and Q(1 – 3k, 3) is
- k.
- mid-point of PQ, using the value of ‘k’ found in (i).
The line through A(–2, 3) and B(4, b) is perpendicular to the line 2x – 4y = 5. Find the value of b.
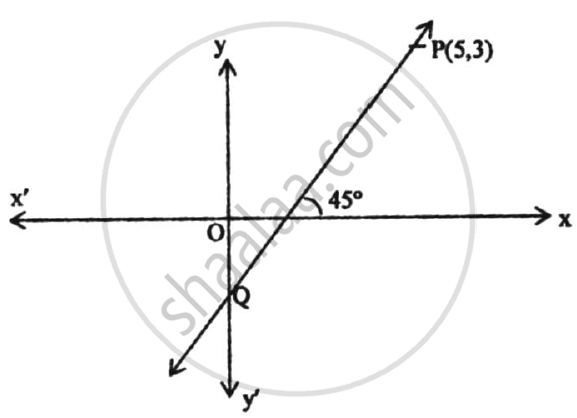
The line through P(5, 3) intersects y-axis at Q.
(1) Write the slope of the line.
(2) Write the equation of the line.
(3) Find the coordinates of Q.
Determine x so that the slope of the line through (1, 4) and (x, 2) is 2.
Lines 2x – by + 5 = 0 and ax + 3y = 2 are parallel to each other. Find the relation connecting a and b.
Find the slope of the lines passing through the given point.
A(2, 3), B(4, 7)
Determine whether the following point is collinear.
L(2, 5), M(3, 3), N(5, 1)
Find k, if B(k, –5), C (1, 2) and slope of the line is 7.
Fill in the blank using correct alternative.
A line makes an angle of 30° with the positive direction of X– axis. So the slope of the line is ______.
Show that points P(1, –2), Q(5, 2), R(3, –1), S(–1, –5) are the vertices of a parallelogram.
Find the type of the quadrilateral if points A(–4, –2), B(–3, –7) C(3, –2) and D(2, 3) are joined serially.
Find the slope of a line, correct of two decimals, whose inclination is 50°
Find the slope of a line passing through the given pair of points (2,5) and (-1,8)
Find slope of a line passing through the points A(3, 1) and B(5, 3).
Verify whether the following points are collinear or not:
A(1, –3), B(2, –5), C(–4, 7).
Find the slope of the line passing through the points A(4,7) and B(2,3).
Find the value of x so that the line passing through (3, 4) and (x, 5) makes an angle 135° with positive direction of X-axis.
If the lines 7y = ax + 4 and 2y = 3 − x, are parallel to each other, then the value of ‘a’ is:
