Advertisements
Advertisements
Question
If ∆ABC and ∆DBC are on the same base BC, AB = DC and AC = DB (see figure), then which of the following gives a congruence relationship?
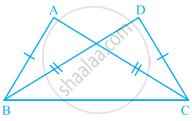
Options
∆ABC ≅ ∆DBC
∆ABC ≅ ∆CBD
∆ABC ≅ ∆DCB
∆ABC ≅ ∆BCD
Solution
∆ABC ≅ ∆DCB
Explanation:
In ∆ABC and ∆DCB,
AB = DC ......(Given)
AC = DB ......(Given)
BC = CB ......(Common)
∴ ∆ABC ≅ ∆DCB ......(SSS criterion)
APPEARS IN
RELATED QUESTIONS
Consider the given pairs of triangles and say whether each pair is that of congruent triangles. If the triangles are congruent, say ‘how’; if they are not congruent say ‘why’ and also say if a small modification would make them congruent: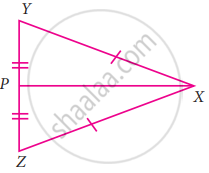
Consider the given pairs of triangles and say whether each pair is that of congruent triangles. If the triangles are congruent, say ‘how’; if they are not congruent say ‘why’ and also say if a small modification would make them congruent: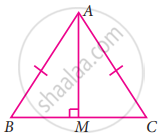
State whether the two triangles are congruent or not. Justify your answer
Construct a triangle XYZ with the given conditions.
XY = 6.4 cm, ZY = 7.7 cm and XZ = 5 cm
Construct a triangle XYZ with the given conditions.
An equilateral triangle of side 7.5 cm
If D is the mid-point of the side BC in ∆ABC where AB = AC, then ∠ADC is ______.
By which congruency criterion, the two triangles in the given figure are congruent?
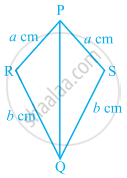
In ∆ABC, AB = 3.5 cm, AC = 5 cm, BC = 6 cm and in ∆PQR, PR = 3.5 cm, PQ = 5 cm, RQ = 6 cm. Then ∆ABC ≅ ∆PQR.
In the following pairs of triangles of figure, the lengths of the sides are indicated along the sides. By applying SSS congruence criterion, determine which triangles are congruent. If congruent, write the results in symbolic form.
ABC is an isosceles triangle with AB = AC and D is the mid-point of base BC (see figure).
- State three pairs of equal parts in the triangles ABD and ACD.
- Is ∆ABD ≅ ∆ACD. If so why?

