Advertisements
Advertisements
Question
If BM and CN are the perpendiculars drawn on the sides AC and AB of the triangle ABC, prove that the points B, C, M and N are concyclic.
Solution
Let O be the center of BC.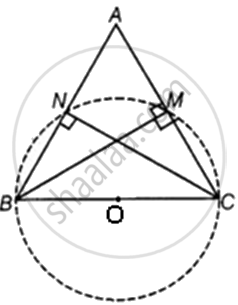
Since CN is perpendicular on AB, therefore ΔBNC is right-angled triangle.
Therefore the circle passing through B, N and C will have midpoint of BC as center and OB be the radius. ...(1)
Similarly, BM is perpendicular on AC, therefore ΔBMC is right-angled triangle.
Therefore the circle passing through B, M and C will have midpoint of BC as center and OB be the radius ...(2)
From (1), we get a circle passing through B, N and C which is centered at O and has radius OB.
From (2), we get a circle passing through B, M and C which is centered at O and has radius OB.
Since from a fixed point and fixed radius, only one circle can be drawn. Therefore, same circle will pass through the four points B, M, N and C.
Therefore; B, N, M and C are concyclic.
APPEARS IN
RELATED QUESTIONS
A line segment AB is of length 5 cm. Draw a circle of radius 4 cm passing through A and B. Can you draw a circle of radius 2 cm passing through A and B? Give reason in support of your answer.
The lengths of two parallel chords of a circle are 6 cm and 8 cm. if the smaller chord is at a distance of 4 cm from the centre, what is the distance of the other chord from the centre?
Three girls Ishita, Isha and Nisha are playing a game by standing on a circle of radius 20 m drawn in a park. Ishita throws a ball o Isha, Isha to Nisha and Nisha to Ishita. If the distance between Ishita and Isha and between Isha and Nisha is 24 m each, what is the distance between Ishita and Nisha.
A chord of a circle is equal to the radius of the circle. Find the angle subtended by the chord at a point on the minor arc and also at a point on the major arc.
If the perpendicular bisector of a chord AB of a circle PXAQBY intersects the circle at P and Q, prove that arc PXA ≅ arc PYB.
A, B and C are three points on a circle. Prove that the perpendicular bisectors of AB, BC and CA are concurrent.
Two circles with centres O and O′ intersect at two points A and B. A line PQ is drawn parallel to OO′ through A(or B) intersecting the circles at P and Q. Prove that PQ = 2 OO′.
