Advertisements
Advertisements
Question
If tan θ = `"m"/"n"`, show that `"m sin θ - n cos θ"/"m sinθ + n cos θ" = ("m"^2 - "n"^2)/("m"^2 + "n"^2)`
Solution
tan θ = `"m"/"n" = "Perpendicular"/"Base"`
Hypotenuse
= `sqrt(("Perpendicular")^2 + ("Base")^2`
= `sqrt("m"^2 + "n"^2`
sin θ = `("m"/sqrt("m"^2 + "n"^2))`
cos θ = `("n"/sqrt("m"^2 + "n"^2))`
To show: `"m sin θ - n cos θ"/"m sinθ + n cos θ" = ("m"^2 - "n"^2)/("m"^2 + "n"^2)`.
`"m sin θ - n cos θ"/"m sinθ + n cos θ"`
= `(("m"/sqrt("m"^2 + "n"^2)) - "n"("n"/sqrt("m"^2 + "n"^2)))/(("m"/sqrt("m"^2 + "n"^2)) + "n"("n"/sqrt("m"^2 + "n"^2))`
= `(("m"^2 - "n"^2)/(sqrt("m"^2 + "n"^2)))/(("m"^2 + "n"^2)/(sqrt("m"^2 + "n"^2)`
= `("m"^2 - "n"^2)/(sqrt("m"^2 + "n"^2)) xx sqrt("m"^2 + "n"^2)/("m"^2 + "n"^2)`
= `("m"^2 - "n"^2)/("m"^2 + "n"^2)`.
APPEARS IN
RELATED QUESTIONS
In the given figure, ∠Q = 90°, PS is a median om QR from P, and RT divides PQ in the ratio 1 : 2. Find: `("tan" ∠"TSQ")/("tan"∠"PRQ")`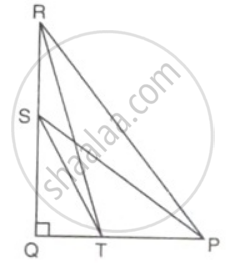
In the given figure, AD is perpendicular to BC. Find: 15 tan y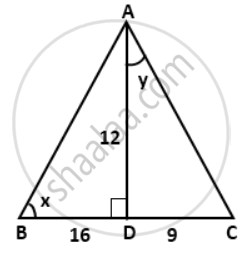
In the given figure, AD is perpendicular to BC. Find:
`(3)/("sin" x) + (4)/("cos" y) - 4 "tan" y`
If 24cosθ = 7 sinθ, find sinθ + cosθ.
If 5cosθ = 3, find the value of `(4cosθ - sinθ)/(2cosθ + sinθ)`
If 4sinθ = `sqrt(13)`, find the value of 4sin3θ - 3sinθ
If cotθ = `(1)/sqrt(3)`, show that `(1 - cos^2θ)/(2 - sin^2θ) = (3)/(5)`
If 12cosecθ = 13, find the value of `(sin^2θ - cos^2θ) /(2sinθ cosθ) xx (1)/tan^2θ`.
If 12 cotθ = 13, find the value of `(2sinθ cosθ)/(cos^2θ - sin^2θ)`.
If secA = `(5)/(4)`, cerify that `(3sin"A" - 4sin^3"A")/(4cos^3"A" - 3cos"A") = (3tan"A" - tan^3"A")/(1 - 3tan^2"A")`.
