Advertisements
Advertisements
Question
In a parallelogram PQRS, M and N are the midpoints of the opposite sides PQ and RS respectively. Prove that
PMRN is a parallelogram.
Solution
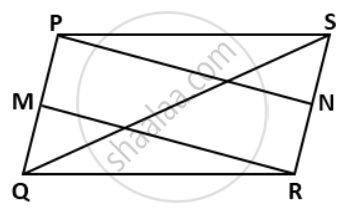
Since M and N are the mid-points of PQ and RS respectively.
∴ PM = `(1)/(2)"PQ" and "RN" = (1)/(2)"RS"` ....(i)
But PQRS is a parallelogram,
∴ PQ = RS and PQ || RS
⇒ `(1)/(2)"PQ" = (1)/(2)"RS" and "PQ || RS"`
⇒ PM = RN and PM || RN
⇒ PMRN is a parallelogram.
APPEARS IN
RELATED QUESTIONS
SN and QM are perpendiculars to the diagonal PR of parallelogram PQRS.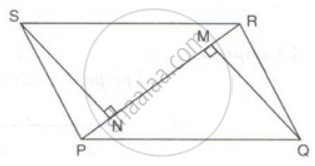
Prove that:
(i) ΔSNR ≅ ΔQMP
(ii) SN = QM
ABCD is a parallelogram. P and T are points on AB and DC respectively and AP = CT. Prove that PT and BD bisect each other.
ABCD is a quadrilateral P, Q, R and S are the mid-points of AB, BC, CD and AD. Prove that PQRS is a parallelogram.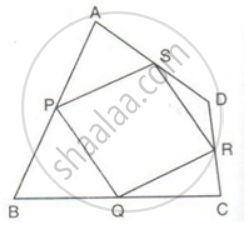
PQRS is a parallelogram. T is the mid-point of RS and M is a point on the diagonal PR such that MR = `(1)/(4)"PR"`. TM is joined and extended to cut QR at N. Prove that QN = RN.
In a parallelogram PQRS, M and N are the midpoints of the opposite sides PQ and RS respectively. Prove that
RN and RM trisect QS.
In a parallelogram PQRS, M and N are the midpoints of the opposite sides PQ and RS respectively. Prove that
MN bisects QS.
Prove that the diagonals of a kite intersect each other at right angles.
Prove that the diagonals of a square are equal and perpendicular to each other.
The diagonals AC and BC of a quadrilateral ABCD intersect at O. Prove that if BO = OD, then areas of ΔABC an ΔADC area equal.
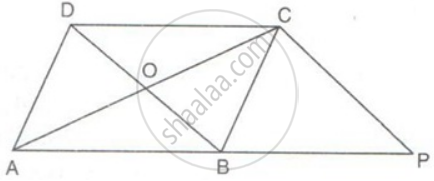
In the figure, ABCD is a parallelogram and CP is parallel to DB. Prove that: Area of OBPC = `(3)/(4)"area of ABCD"`