Advertisements
Advertisements
Question
In a triangle ABC, AB = 4 cm, BC = 4.5 cm and CA = 5 cm. Construct ΔABC. Find the image A'B'C of the ΔABC obtained by enlarging it by a scale factor 2. Measure the sides of the image A'B'C' and show that AB:A'B' = AC:B'C' = CA:C'A'
Solution

Steps of COnstruction of the image: :
1. Draw BC measuring 4cm.
2. With B as the centre and radius 4.5cm, make an arc above BC.
3. With C as the centre and radius 5cm, to cut the previous arc at C.
4. ΔABC us the required triangle.
Scale factor = `"A'B'"/"AB"`
⇒ 2 = `"A'B'"/(4)`
⇒ A'B' = 8cm
Scale factor = `"B'C'"/"BC"`
⇒ 2 = `"B'C'"/(4.5)`
⇒ B'C' = 9cm
Scale factor = `"A'C'"/"AC"`
⇒ 2 = `"A'C'"/(5)`
⇒ A'C' = 10cm
Steps of Construction of the image: :
1. Draw B'C' measuring 9cm.
2. With B' as the centre and radius 8cm, make an arc above B'C'.
3. With C' as the centre and radius 9cm, to cut the pervious arc at C'.
4. ΔA'B'C' is the required image of the ΔABC.
On measuring the sides, we get
`"A'B'"/"AB" = "B'C'"/"BC" = "A'C'"/"AC"` = Scale factor = 2.
APPEARS IN
RELATED QUESTIONS
See the given figure. DE || BC. Find EC.
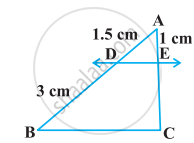
In the given figure, AB and DE are perpendicular to BC.

1) Prove that ΔABC ∼ ΔDEC
2) If AB = 6 cm; DE = 4 cm and AC = 15 cm. Calculate CD.
3) Find the ratio of area of ΔABC: area of ΔDEC
In triangle ABC, AD is perpendicular to side BC and AD2 = BD × DC. Show that angle BAC = 90°.
The given figure shows a triangle PQR in which XY is parallel to QR. If PX : XQ = 1 : 3 and QR = 9 cm, find the length of XY.

Further, if the area of ΔPXY = x cm2; find, in terms of x the area of :
- triangle PQR.
- trapezium XQRY.
In the given figure, ∠PQR = ∠PST = 90° ,PQ = 5cm and PS = 2cm.
(i) Prove that ΔPQR ~ ΔPST.
(ii) Find Area of ΔPQR : Area of quadrilateral SRQT.

In the figure , ABCD is a quadrilateral . F is a point on AD such that AF = 2.1 cm and FD = 4.9 cm . E and G are points on AC and AB respectively such that EF || CD and GE || BC . Find `("Ar" triangle "BCD")/("Ar" triangle "GEF")`

In the following figure, M is mid-point of BC of a parallelogram ABCD. DM intersects the diagonal AC at P and AB produced at E. Prove that : PE = 2 PD

The areas of two similar triangles are 16cm2 and 9cm2 respectively. If the altitude of the smaller triangle is 1.8cm, find the length of the altitude corresponding to the larger triangle.
A model of a ship is made to a scale of 1:500. Find: The volume of the model when the volume of the ship is 1km3
Two triangles are similar. Smaller triangle’s sides are 4 cm, 5 cm, 6 cm. Perimeter of larger triangle is 90 cm then find the sides of larger triangle.
