Advertisements
Advertisements
Question
In the figure , ABCD is a quadrilateral . F is a point on AD such that AF = 2.1 cm and FD = 4.9 cm . E and G are points on AC and AB respectively such that EF || CD and GE || BC . Find `("Ar" triangle "BCD")/("Ar" triangle "GEF")`

Solution
In Δ ABC, GE || BC
∴ By BPT
`"AG"/"GB" = "AF"/"FD"` .......(1)
Similarly, in Δ ACD
`"AE"/"EC" = "AF"/"FD"` .....(2)
From ( 1) and (2)
`"AG"/"GB" = "AF"/"FD"`
∴ GE || BC ...(By converse of BPT)
In Δ AGF and Δ ABD
∠ A = ∠ A (common)
∠ AFG = ∠ ADB (Corresponding angles)
∴ Δ AGF ∼ Δ ABD (AA corollary)
∴ `"AF"/"AD" = "GF"/"BD"` (Similar sides of similar triangles)
`2.1/7 = "GF"/"BD"`
`3/10 = "GF"/"BD"`
`("Ar" triangle "GEF")/("Ar" triangle "BCD") = "GF"^2/"BD"^2`
[The ration of areas of two similar triangle is equal to the ratio of square of their corresponding sides.]
= `(3/10)^2`
= `9/100`
= 9 : 100
`("Ar" triangle "BCD") : ("Ar" triangle "GEF")` = 100 : 9
APPEARS IN
RELATED QUESTIONS
Prove that, in a right triangle, the square on the hypotenuse is equal to the sum of the squares on the other two sides.
In the given figure, ∆ABC and ∆AMP are right angled at B and M respectively.
Given AC = 10 cm, AP = 15 cm and PM = 12 cm.
- Prove that: ∆ABC ~ ∆AMP
- Find: AB and BC.

In an isosceles ΔABC, the base AB is produced both ways in P and Q such that
AP × BQ = AC2.
Prove that ΔACP~ΔBCQ.

ABCD and PQRS are similar figures. AB= 12cm, BC=x cm, CD= 15 cm, AD= 10 cm, PQ= 8 cm, QR = 5 cm, RS = m cm and PS = n cm .Find the values of x, m and n.
Let ∆ ABC ∽ ∆ DEF and their areas be respectively, 64 cm2 and 121 cm2. If EF = 15⋅4 cm, find BC.
In the figure, PR || SQ. If PR = 10cm, PT = 5cm, TQ = 6cm and ST = 9cm, calculate RT and SQ.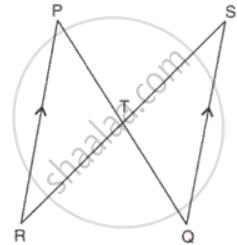
On a map drawn to a scale of 1:25000, a rectangular plot of land has sides 12cm x 16cm. Calculate: The area of the plot in sq km
Check whether the triangles are similar and find the value of x
A man whose eye-level is 2 m above the ground wishes to find the height of a tree. He places a mirror horizontally on the ground 20 m from the tree and finds that if he stands at a point C which is 4 m from the mirror B, he can see the reflection of the top of the tree. How height is the tree?
In ΔABC, AP ⊥ BC and BQ ⊥ AC, B−P−C, A−Q−C, then show that ΔCPA ~ ΔCQB. If AP = 7, BQ = 8, BC = 12, then AC = ?

In ΔCPA and ΔCQB
∠CPA ≅ [∠ ______] ...[each 90°]
∠ACP ≅ [∠ ______] ...[common angle]
ΔCPA ~ ΔCQB ......[______ similarity test]
`"AP"/"BQ" = (["______"])/"BC"` .......[corresponding sides of similar triangles]
`7/8 = (["______"])/12`
AC × [______] = 7 × 12
AC = 10.5
