Advertisements
Advertisements
Question
A man whose eye-level is 2 m above the ground wishes to find the height of a tree. He places a mirror horizontally on the ground 20 m from the tree and finds that if he stands at a point C which is 4 m from the mirror B, he can see the reflection of the top of the tree. How height is the tree?
Solution
Let the height of the tree AD be “h”.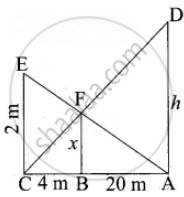
In ∆ACD and ∆BCF,
∠A = ∠B = 90°
∠C is common
∆ACD ~ ∆BCF by AA similarity
`"AD"/"BF" = "AC"/"BC"`
`"h"/x = 24/2` = 6
h = 6x ...(1)
In ∆ACE and ∆ABF,
∠C = ∠B = 90°
∠A is common
∴ ∆ACE ~ ∆ABF
`"CE"/"BF" = "AC"/"AB"`
`2/x = 24/20`
24x = 20 × 2
x = `(20 xx 2)/24 = (5 xx 2)/6 = 10/6`
x = `5/3`
Substitute the value of x in (1)
h = `6 xx 5/3` = 10 m
∴ Height of the tree is 10 m
APPEARS IN
RELATED QUESTIONS
In figure, if ∠A = ∠C, then prove that ∆AOB ~ ∆COD

In ΔPQR, MN is parallel to QR and `(PM)/(MQ) = 2/3`
1) Find `(MN)/(QR)`
2) Prove that ΔOMN and ΔORQ are similar.
3) Find, Area of ΔOMN : Area of ΔORQ

P and Q are points on the sides AB and AC respectively of a ΔABC. If AP = 2cm, PB = 4cm, AQ = 3cm and QC = 6cm, show that BC = 3PQ.
In the following figure, point D divides AB in the ratio 3 : 5. Find : `(AD)/(AB)`

In the given figure, ΔABC ~ ΔADE. If AE : EC = 4 : 7 and DE = 6.6 cm, find BC. If 'x' be the length of the perpendicular from A to DE, find the length of perpendicular from A to BC in terms of 'x'.

D and E are points on the sides AB and AC respectively of a Δ ABC such that DE | | BC and divides Δ ABC into two parts, equal in area. Find `"BD"/"AB"`.
If ΔABC, D and E are points on AB and AC. Show that DE || BC for each of the following case or not:
AB = 5.6cm, AD = 1.4cm, AC = 7.2cm, and AE = 1.8cm
The areas of two similar triangles are 16cm2 and 9cm2 respectively. If the altitude of the smaller triangle is 1.8cm, find the length of the altitude corresponding to the larger triangle.
The scale of a map is 1 : 50000. The area of a city is 40 sq km which is to be represented on the map. Find: The area of land represented on the map.
In the given figure the value of x is

