Advertisements
Advertisements
Question
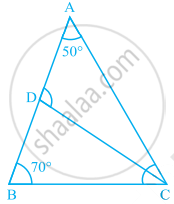
In ∆ABC, ∠Α = 50°, ∠B = 70° and bisector of ∠C meets AB in D (see figure). Measure of ∠ADC is ______.
Options
50°
100°
30°
70°
Solution
In ∆ABC, ∠Α = 50°, ∠B = 70° and bisector of ∠C meets AB in D (see figure). Measure of ∠ADC is 100°.
Explanation:

In ∆ABC,
∠ADC + ∠DAC + ∠ACD = 180° ......[Angle sum property of a triangle]
⇒ ∠ADC + 50° + ∠ACD = 180° ......[∵ ∠DAC = 50°]
⇒ ∠ACD = 130° – ∠ADC ......(i)
In ∆DBC, ∠ADC = ∠DBC + ∠BCD ......[∵ Exterior angle is equal to the sum of opposite interior angles]
⇒ ∠ADC = 70° + ∠ACD ......[∵ ∠ACD = ∠BCD]
⇒ ∠ADC = 70° + 130° – ∠ADC ......[From equation (i)]
⇒ ∠ADC = 200° – ∠ADC
⇒ 2∠ADC = 200°
⇒ ∠ADC = `200^circ/2`
⇒ ∠ADC = 100°
APPEARS IN
RELATED QUESTIONS
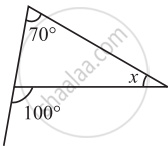
Find the value of the unknown exterior angle x in the following diagram:

Find the value of the unknown interior angle x in the following figure.
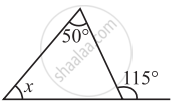
Find the value of the unknown interior angle x in the following figure.
If the exterior angle of a triangle is 140° and its interior opposite angles are equal, find all the interior angles of the triangle
In the figure find the value of x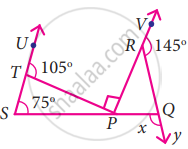
In ∆PQR, if ∠P = 60°, and ∠Q = 40°, then the exterior angle formed by producing QR is equal to ______.
The sum of an exterior angle of a triangle and its adjacent angle is always ______.
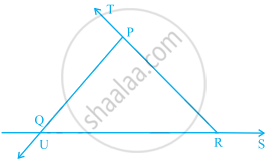
In the given figure, ∠TPQ = ∠ _____ + ∠ _____
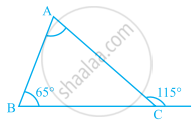
Find the measure of ∠A in the given figure.
In the given figure, ∆PQR is right-angled at P. U and T are the points on line QRF. If OP || ST and US || RP, find ∠S.

