Advertisements
Advertisements
Question
Find the value of the unknown interior angle x in the following figure.
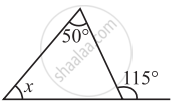
Solution
x + 50° = 115° (Exterior angle property)
x = 115° − 50°
x = 65°
APPEARS IN
RELATED QUESTIONS
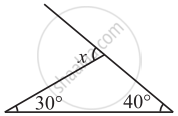
Find the value of the unknown exterior angle x in the following diagram:
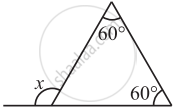
Find the value of the unknown exterior angle x in the following diagram:
In the isosceles triangle ABC, ∠A, and ∠B are equal. ∠ACD is an exterior angle of ∆ABC. The measures of ∠ACB and ∠ACD are (3x − 17)° and (8x + 10)°, respectively. Find the measures of ∠ACB and ∠ACD. Also find the measures of ∠A and ∠B.
An exterior angle of a triangle is 70° and two interior opposite angles are equal. Then measure of each of these angle will be
In the given figure BD = BC, find the value of x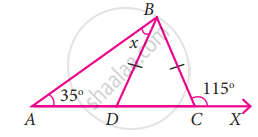
In the given figure, BC = CA and ∠A = 40°. Then, ∠ACD is equal to ______.
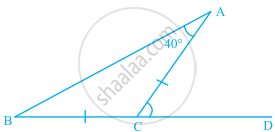
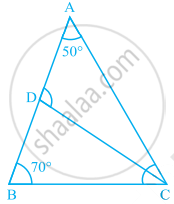
In ∆ABC, ∠Α = 50°, ∠B = 70° and bisector of ∠C meets AB in D (see figure). Measure of ∠ADC is ______.
The sum of an exterior angle of a triangle and its adjacent angle is always ______.
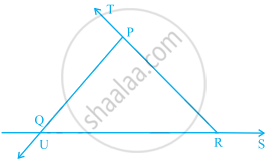
In the given figure, ∠TPQ = ∠ _____ + ∠ _____
In ∆ABC, if ∠A = ∠C, and exterior angle ABX = 140°, then find the angles of the triangle.
