Advertisements
Advertisements
Question
In the below fig. ABCD is a trapezium in which AB = 7 cm, AD = BC = 5 cm, DC = x cm,
and distance between AB and DC is 4cm. Find the value of x and area of trapezium ABCD.
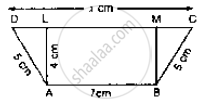
Solution
Draw AL ⊥ DC, BM ⊥ DC Then ,
AL = BM = 4cm and LM = 7 cm
In ΔADL, we have
AD2 = AL2 + DL2 ⇒ 25 = 16 + DL2 ⇒ DL= 3 cm
`Similarly MC = sqrt(BC^2-BM^2)=sqrt(25-16)=3cm`
∴ x = CD = CM + ML + CD = 3 + 7 + 3 = 13cm
`ar (trap . ABCD ) = 1/2 ( AB + CD) × AL 1/2 (7 + 13) xx 4cm^2`
= 4cm2
APPEARS IN
RELATED QUESTIONS
In Q. No 1, if AD = 6 cm, CF = 10 cm, and AE = 8cm, find AB.
If ABCD is a parallelogram, then prove that
๐๐ (Δ๐ด๐ต๐ท) = ๐๐ (Δ๐ต๐ถ๐ท) = ๐๐ (Δ๐ด๐ต๐ถ) = ๐๐ (Δ๐ด๐ถ๐ท) = `1/2` ๐๐ (||๐๐ ๐ด๐ต๐ถ๐ท) .
If AD is a median of a triangle ABC, then prove that triangles ADB and ADC are equal in
area. If G is the mid-point of median AD, prove that ar (Δ BGC) = 2 ar (Δ AGC).
In square ABCD, P and Q are mid-point of AB and CD respectively. If AB = 8cm and PQand BD intersect at O, then find area of ΔOPB.
Let ABC be a triangle of area 24 sq. units and PQR be the triangle formed by the mid-points of the sides of Δ ABC. Then the area of ΔPQR is
Find the area of a rectangle whose length = 15 cm breadth = 6.4 cm
The side of a square is 3.6 cm; find its area.
By counting squares, estimate the area of the figure.
The King was very happy with carpenters Cheggu and Anar. They had made a very big and beautiful bed for him. So as gifts the king wanted to give some land to Cheggu, and some gold to Anar. Cheggu was happy. He took 100 meters of wire and tried to make different rectangles.
He made a 10 m × 40 m rectangle. Its area was 400 square meters. So he next made a 30 m × 20 m rectangle.
- What is its area? Is it more than the first rectangle?
Whose footprint is larger - yours or your friend’s?
