Advertisements
Advertisements
Question
If AD is a median of a triangle ABC, then prove that triangles ADB and ADC are equal in
area. If G is the mid-point of median AD, prove that ar (Δ BGC) = 2 ar (Δ AGC).
Solution

Draw AM ⊥ BC
Since, AD is the median of ΔABC
∴ BD = DC
⇒ BD = AM = DC × AM
⇒ ` 1/2 (BD xx AM ) = 1/2 ( DC xx AM)`
⇒ ar (Δ ABC) = ar (Δ ACD) ........ (1)
In ΔBGC , GDis the median
∴ ar (BGD) = area (OGD) ......... (2)
In ΔACD , CG is the median
∴ area (AGC) = area (Δ CGD) ......... (3)
From (1) and (2) , we have
Area (ΔBGD) = ar (Δ AGC)
But, ar (ΔBGC) = 2ar (BGD)
∴ ar (BGC ) = 2ar (Δ AGC)
APPEARS IN
RELATED QUESTIONS
ABCD is a parallelogram. P is the mid-point of AB. BD and CP intersect at Q such that CQ: QP = 3.1. If ar (ΔPBQ) = 10cm2, find the area of parallelogram ABCD.
The area of the figure formed by joining the mid-points of the adjacent sides of a rhombus with diagonals 16 cm and 12 cm is
The sides of a rectangular park are in the ratio 4 : 3. If its area is 1728 m2, find
(i) its perimeter
(ii) cost of fencing it at the rate of ₹40 per meter.
Find the area of a square, whose side is: 4.1 cm.
Who had the bigger piece? How much bigger?
Look at the table. If you were to write the area of each of these which column would you choose? Make a (✓).
| Square cm |
Square meter |
Square km |
|
| Handkerchief | ✓ | ||
| Sari | |||
| Page of your book | |||
| School land | |||
| Total land of a city | |||
| Door of your classroom | |||
| Chair seat | |||
| Blackboard | |||
| Indian flag | |||
| Land over which a river flows |
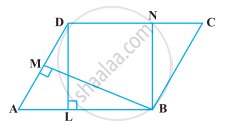
In the following figure, the area of parallelogram ABCD is ______.
Area of a rectangle with length 5 cm and breadth 3 cm is ______.
Find the area of the following figure by counting squares:

Find the area of the following figure by counting squares:

