Advertisements
Advertisements
Question
In the below fig, lines AB, CD and EF intersect at O. Find the measures of ∠AOC, ∠COF,
∠DOE and ∠BOF.
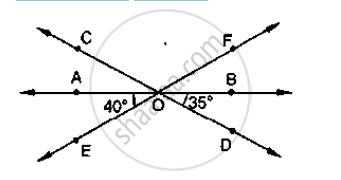
Solution
`∠`AOE and `∠`EOB are linear pair of angles
`∠`AOE + `∠`EOB = 180°
`∠`AOE + `∠`DOE + `∠`BOD = 180°
⇒ `∠`DOE = 180° - 40° - 35° = 105°
Vertically opposite side angles are equal
`∠`DOE = `∠`COF = 105°
Now, `∠`AOE + `∠`AOF = 180° [∵ Linear pair]
⇒ `∠`AOE + `∠`AOC + `∠`COF = 180°
⇒ 40° + `∠`AOC +105° = 180°
⇒ `∠`AOC = 180° -145°
⇒ `∠`AOC = 35°
Also, `∠`BOF = `∠`AOE = 40° [ ∵ Vertically opposite angle are equal]
APPEARS IN
RELATED QUESTIONS
How many pairs of adjacent angles are formed when two lines intersect in a point?
In the below fig, three coplanar lines intersect at a point O, forming angles as shown in the figure. Find the values of x, y, z and u.

Prove that the bisectors of a pair of vertically opposite angles are in the same straight line.
If l, m, n are three lines such that l || m and n ⊥ l, prove that n ⊥ m.
Given ∠POR = 3x and ∠QOR = 2x + 10°. If POQ is a straight line, then the value of x is
In the given figure, AOB is a straight line. If ∠AOC + ∠BOD = 85°, then ∠COD =
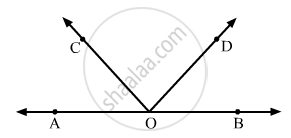
In the given figure, the value of y is
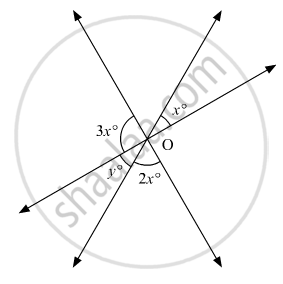
In the given figure, the value of x is ______.

Two lines are respectively perpendicular to two parallel lines. Show that they are parallel to each other.
Two lines in a plane which do not meet at a point anywhere are called ______ lines.
