Advertisements
Advertisements
Question
Prove that the bisectors of a pair of vertically opposite angles are in the same straight line.
Solution
Given,
Lines AOB and COD intersect at point O such that
`∠`AOC = `∠`BOD
Also OE is the bisector `∠`ADC and OF is the bisector `∠`BOD
To prove: EOF is a straight line vertically opposite angles is equal
`∠`AOD = `∠`BOC = 5x .......(1)
Also `∠`AOC + `∠`BOD
⇒ 2`∠`AOE = 2`∠`DOF .......(2)
Sum of the angles around a point is 360°
⇒ 2`∠`AOD + 2`∠`AOE + 2`∠`DOF = 360°
⇒`∠`AOD + `∠`AOF + `∠`DOF = 180°
From this we conclude that EOF is a straight line.
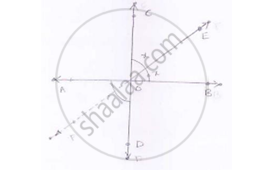
Given that :- AB and CD intersect each other at O
OE bisects `∠`COB
To prove: `∠`AOF = `∠`DOF
Proof: OE bisects `∠`COB
`∠`COE = `∠`EOB = x
Vertically opposite angles are equal
`∠`BOE = `∠`AOF = x .......(1)
`∠`COE = `∠`DOF = x .......(2)
From (1) and (2)
`∠`AOF = `∠`DOF = x
APPEARS IN
RELATED QUESTIONS
How many pairs of adjacent angles are formed when two lines intersect in a point?
In the below fig, lines PQ and RS intersect each other at point O. If ∠POR: ∠ROQ − 5 : 7,
find all the angles.

If l, m, n are three lines such that l || m and n ⊥ l, prove that n ⊥ m.
Two straight line AB and CD intersect one another at the point O. If ∠AOC + ∠COB + ∠BOD = 274°, then ∠AOD =
Consider the following statements:
When two straight lines intersect:
(i) adjacent angles are complementary
(ii) adjacent angles are supplementary
(iii) opposite angles are equal
(iv) opposite angles are supplementary
Of these statements
In the given figure, the value of x is ______.

Two lines AB and CD intersect at O. If ∠AOC + ∠COB + ∠BOD = 270°, then ∠AOC =
Give two examples of perpendicular lines you can see in your environment.
Two lines are respectively perpendicular to two parallel lines. Show that they are parallel to each other.
Two parallel lines meet each other at some point.
