Advertisements
Advertisements
Question
If two straight lines intersect each other, prove that the ray opposite to the bisector of one of the angles thus formed bisects the vertically opposite angle.
Solution
Let AB and CD intersect at a point O
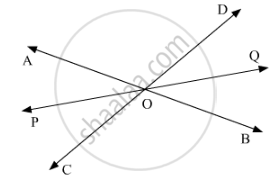
Also, let us draw the bisector OP of ∠AOC.
Therefore,
∠AOP = ∠POC (1)
Also, let’s extend OP to Q.
We need to show that, OQ bisects ∠BOD.
Let us assume that OQ bisects∠BOD, now we shall prove that POQ is a line.
We know that,
∠AOCand ∠DOBare vertically opposite angles. Therefore, these must be equal, that is:
∠AOC = ∠DOB (2)
∠AOPand ∠BOQ are vertically opposite angles. Therefore,
∠AOP = ∠BOQ
Similarly,
∠POC = ∠DOQ
We know that:
∠AOP +∠AOD+∠DOQ+∠POC+∠BOC+∠BOQ = 360°
2∠AOP+∠AOD+2∠DOQ+∠BOC =360°
2∠AOP + 2∠AOD+ 2∠DOQ = 360°
2(∠AOP+∠AOD+ ∠DOQ) = 360°
∠AOP+∠AOD+ ∠DOQ = `(360°)/2`
∠AOP+∠AOD+ ∠DOQ = 180°
Thus, POQ is a straight line.
Hence our assumption is correct. That is,
We can say that if the two straight lines intersect each other, then the ray opposite to the bisector of one of the angles thus formed bisects the vertically opposite angles.
APPEARS IN
RELATED QUESTIONS
If an angle is 30° more than one half of its complement, find the measure of the angle.
One angle is equal to three times its supplement. The measure of the angle is
State, true or false:
An infinite number of straight lines can be drawn through a given point.
How many lines can be drawn through three
(a) collinear points?
(b) non-collinear points?
In the following figure, ∠AOB and ∠AOC are adjacent angles? Give the reason for your answer.

Write the complement of 90°
Write the complement of `1/2` of a right angle
Write the supplement of (90 + a + b)°
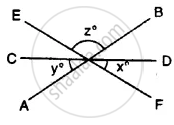
AB, CD and EF are three lines intersecting at the same point.
(i) Find x, if y = 45° and z = 90°.
(ii) Find a, if x = 3a, y = 5x and r = 6x.
In the given figure, the arrows indicate parallel lines. State which angles are equal. Give a reason.

