Advertisements
Advertisements
Question
If l, m, n are three lines such that l || m and n ⊥ l, prove that n ⊥ m.
Solution

Given l || , m ,n perpendicular l
To prove: n ⊥ m
Since l || m and n intersects them at G and H respectively
∴
But, U = 90° [n ⊥ l ]
⇒
Hence n perpendicular m
APPEARS IN
RELATED QUESTIONS
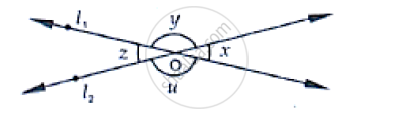
In the below fig, lines �1 and �2 intersect at O, forming angles as shown in the figure. If x = 45, Find the values of x, y, z and u.
Prove that the bisectors of a pair of vertically opposite angles are in the same straight line.
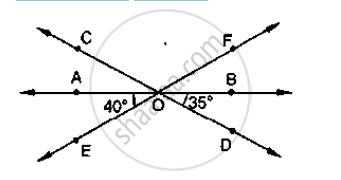
In the below fig, lines AB, CD and EF intersect at O. Find the measures of ∠AOC, ∠COF,
∠DOE and ∠BOF.
Two straight line AB and CD intersect one another at the point O. If ∠AOC + ∠COB + ∠BOD = 274°, then ∠AOD =
Consider the following statements:
When two straight lines intersect:
(i) adjacent angles are complementary
(ii) adjacent angles are supplementary
(iii) opposite angles are equal
(iv) opposite angles are supplementary
Of these statements
Given ∠POR = 3x and ∠QOR = 2x + 10°. If POQ is a straight line, then the value of x is
Two lines AB and CD intersect at O. If ∠AOC + ∠COB + ∠BOD = 270°, then ∠AOC =
Give two examples of parallel lines you can see in your environment.
Look at the picture given below. Decide whether the lines given in picture is parallel or perpendicular to each other and write the answer in the box.

Two parallel lines meet each other at some point.
