Advertisements
Advertisements
Question
In Fig. 6, find the area of the shaded region, enclosed between two concentric circles of radii 7 cm and 14 cm where ∠AOC = 40°. (use `pi = 22/7`)

Solution
Area of the region ABDC = Area of sector AOC – Area of sector BOD
`=40^@/360^@xx22/7xx14xx14-40^@/360^@xx22/7xx7xx7`
`=1/9xx22xx14xx2-1/9xx22xx7xx1`
`=22/9xx(28-7)`
`=22/9xx21`
=`154/3`
=51.33 cm2
Area of circular ring = `22/7xx14xx14-22/7xx7xx7`
= 22x14x2-22x7x1
=22x(28-7)
=22x21
=462 cm2
∴ Required shaded region Area of circular ring Area of region ABDC
= 462 - 51.33
= 410.67 cm2
Thus, the area of shaded region is 410.67 cm2
APPEARS IN
RELATED QUESTIONS
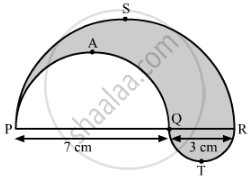
In PSR, RTQ and PAQ are three semicircles of diameters 10 cm, 3 cm and 7 cm respectively. Find the perimeter of the shaded region. [Use π=3.14]
If the circumference of a circular sheet is 154 m, find its radius. Also find the area of
the sheet. (Take `pi = 22/7`)
Saima wants to put a lace on the edge of a circular table cover of diameter 1.5 m. Find the length of the lace required and also find its cost if one meter of the lace costs ₹ 15. (Take π = 3.14)
The minute hand of a circular clock is 15 cm long. How far does the tip of the minute hand move in 1 hour. (Take π = 3.14)
A steel wire when bent is the form of square encloses an area of 12 cm2. If the same wire is bent in form of circle. Find the area of circle.
The area between the circumferences of two concentric circles is 2464 cm2. If the inner circle has circumference of 132 cm, calculate the radius of outer circle.
A horse is tied with a 21 m laig rope to the corner of a field which is in the shape of an equilateral triangle. Find the area of the field over which it can graze.
Find the volume and the surface area of the spheres in the following :
Diameter= 6.3 cm
Sum of the areas of two squares is 157 m2. If the sum of their perimeters is 68 m, find the sides of the two squares.
Find the perimeter of the given shape (Take π = `22/7`).