Advertisements
Advertisements
Question
In the figure given below, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find:
1) AB.
2) the length of tangent PT.

Solution
Theorem used: Product of the lengths of the segments of the chord is equal to the square of the length of the tangent from the point of contact to the point of intersection.
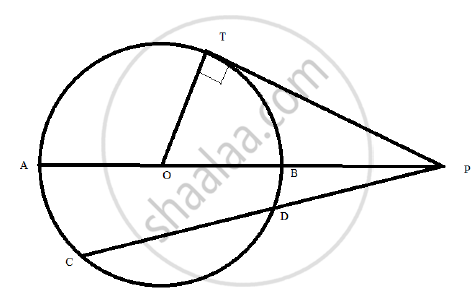
1) As chord CD and tangent at point T intersect each other at P,
`PC xx PD = PT^2` ...(i)
AB is the diameter and tangent at point T intersect each other at P,
`PA xx PB = PT^2` ...(ii)
From (i) and (ii), PC × PD = PA × PB … (iii)
Given: PD = 5cm, CD = 7.8 cm
PA = PB + AB = 4 + AB, and PC = PD + CD = 12.8 cm
Subs. these values in (iii),
12.8 × 5 = (4 + AB) × 4
`=> 4 + AB = (12.8 xx 5)/4`
`=> 4 + AB = 16`
`=> AB = 12cm`
2) `PC xx PD = PT^2`
`=> PT^2 = 12.8 xx 5 =64`
`=> PT = 8 cm`
Thus, the length of the tangent is 8 cm
APPEARS IN
RELATED QUESTIONS
Draw a circle of radius 5 cm. Draw two tangents to this circle so that the angle between the tangents is 45°.
The bisectors of angles A and B of a scalene triangle ABC meet at O.
- What is the point O called?
- OR and OQ are drawn perpendicular to AB and CA respectively. What is the relation between OR and OQ?
- What is the relation between angle ACO and angle BCO?
- Using ruler and compasses only, construct a triangle ABC in which AB = 8 cm, BC = 6 cm and CA = 5 cm.
- Find its in centre and mark it I.
- With I as centre, draw a circle which will cut off 2 cm chords from each side of the triangle. What is the length of the radius of this circle.
Draw a circle with the help of a bangle. Take any point P outside the circle. Construct the pair of tangents form the point P to the circle
Write the steps of construction for drawing a pair of tangents to a circle of radius 3 cm , which are inclined to each other at an angle of 60° .
Using ruler and compasses only, draw tangents to a circle of radius 3 cm from a point 5 cm from the centre. What is the length of each of them ?
Draw two circles of radii 3.5 cm and 2 cm respectively so that their centres are 6 cm apart. Draw direct common tangents to the circle and show that they are equal in length.
Take a point O on the plane at the paper. With O as center draw a circle of radius 3 cm. Take a point P on this circle and draw a tangent at P.
Draw a circle at a radius of 3 cm. Take a point at 5.5 cm from the center at the circle. From point P, draw two tangents to the circle.
A circle of radius r has a center O. What is first step to construct a tangent from a generic point P which is at a distance r from O?
