Advertisements
Advertisements
Question
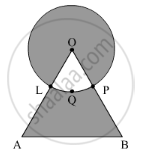
In the given figure, AB and CD are two diameters of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region. [Use Π = 22/7]
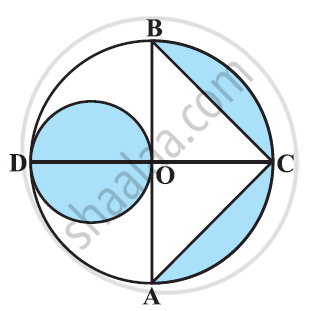
Solution
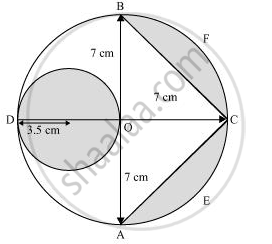
Radius (r1) of larger circle = 7 cm
Radius (r2) of smaller circle = 7/2 cm
Area of smaller circle = `pir_1^2`
`=22/7xx7/2xx7/2`
`= 77/2 cm^2`
Area of semi-circle AECFB of larger circle = `1/2 pir_2^2`
`=1/2xx22/7xx(7)^2`
= 77 cm2
Area of ΔABC = `1/2 xx AB xx OC`
`= 1/2xx14xx7 = 49 cm^2`
Area of the shaded region
= Area of smaller circle + Area of semi-circle AECFB − Area of ΔABC
`= 77/2 + 77 - 49`
`= 28+77/2 = 28 + 38.5 = 66.5 cm^2`
APPEARS IN
RELATED QUESTIONS
The given figure depicts a racing track whose left and right ends are semicircular.
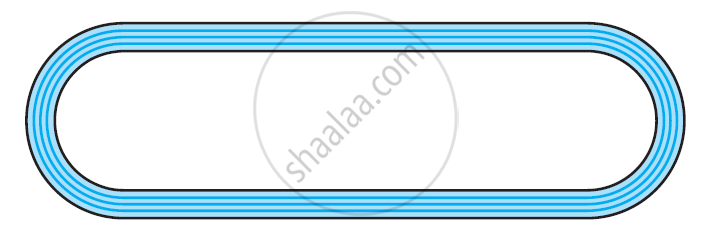
The distance between the two inner parallel line segments is 60 m and they are each 106 m long. If the track is 10 m wide, find:
(i) The distance around the track along its inner edge
(ii) The area of the track
[Use Π = 22/7]
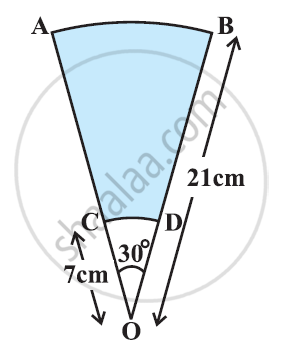
AB and CD are respectively arcs of two concentric circles of radii 21 cm and 7 cm and centre O (see the given figure). If ∠AOB = 30°, find the area of the shaded region. [Use Π = 22/7]
Find the area of triangle whose base measures 24 cm and the corresponding height measure 14.5 cm.
The perimeter of a right triangle is 40 cm and its hypotenuse measure 17 cm. Find the area of the triangle.
Each side of an equilateral triangle is 10 cm. Find (i) the area of the triangle and (ii) the height of the triangle.
If the area of an equilateral triangle is `36sqrt3 cm^2` find its perimeter.
Find the area of shaded region in Fig. 4, where a circle of radius 6 cm has been drawn with vertex O of an equilateral triangle OAB of side 12 cm. (Use π = 3.14 and\[\sqrt{3}\] =1.73)
In Figure 5, ABCD is a quadrant of a circle of radius 28 cm and a semi circle BEC is drawn with BC as diameter. Find the area of the shaded region ?\[[Use\pi = \frac{22}{7}]\]
Find the area of triangle formed by joining the mid-points of the sides of the triangle whose vertices are A(2, 1), B(4, 3) and C(2, 5).
Read the following passage:
Governing council of a local public development authority of Dehradun decided to build an adventurous playground on the top of a hill, which will have adequate space for parking.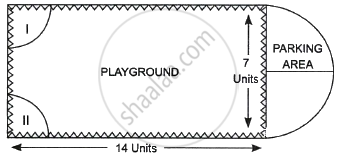 After survey, it was decided to build rectangular playground, with a semi-circular area allotted for parking at one end of the playground. The length and breadth of the rectangular playground are 14 units and 7 units, respectively. There are two quadrants of radius 2 units on one side for special seats. |
Based on the above information, answer the following questions:
- What is the total perimeter of the parking area?
- (a) What is the total area of parking and the two quadrants?
OR
(b) What is the ratio of area of playground to the area of parking area? - Find the cost of fencing the playground and parking area at the rate of ₹ 2 per unit.
