Advertisements
Advertisements
Question
The perimeter of a right triangle is 40 cm and its hypotenuse measure 17 cm. Find the area of the triangle.
Solution
The perimeter of a right-angled triangle = 40 cm
therfore,` a+b+c=40`
Hypotenuse = 17 cm
Therefore, c = 17cm
`a+b+c=40 cm`
⇒`a+b+17=40`
⇒` a+b=23`
⇒`b=23-a ` .................(1)
Now, using Pythagoras theorem, we have:
`a^2+b^2=c^2`
⇒ `a^2+(23-a)^2=17^2`
⇒` a^2+529-46a+a^2=289`
⇒` 2a^2-46a+529-289=0`
⇒`2a^2-46a+240=0`
⇒` a^2-23a+120=0`
⇒`(a-15) (a-8)=0`
⇒a=15 or a=8
Substituting the value of a=15, in equation (i) we get:
`b=23-a`
=`23-15`
=`8 cm`
If we had chosen 8, a cm, then, `b=23-8=15 cm`
In any case,
`"Area of triangle"=1/2xx"Base"xx"height"`
=`1/2xx8xx15`
=`60 cm^2`
APPEARS IN
RELATED QUESTIONS
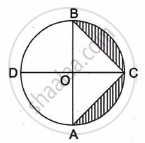
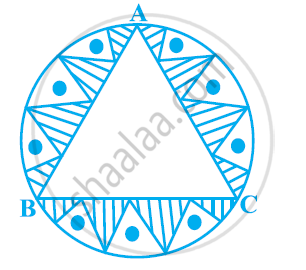
In a circular table cover of radius 32 cm, a design is formed leaving an equilateral triangle ABC in the middle as shown in the given figure. Find the area of the design (Shaded region). [Use Π = 22/7]
The given figure depicts a racing track whose left and right ends are semicircular.
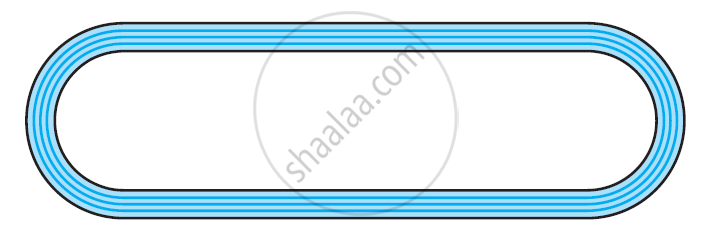
The distance between the two inner parallel line segments is 60 m and they are each 106 m long. If the track is 10 m wide, find:
(i) The distance around the track along its inner edge
(ii) The area of the track
[Use Π = 22/7]
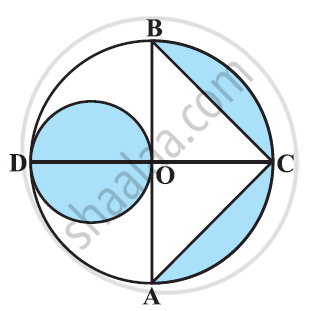
In the given figure, AB and CD are two diameters of a circle (with centre O) perpendicular to each other and OD is the diameter of the smaller circle. If OA = 7 cm, find the area of the shaded region. [Use Π = 22/7]
In the given figure, OACB is a quadrant of circle with centre O and radius 3.5 cm. If OD = 2 cm, find the area of the
(i) Quadrant OACB
(ii) Shaded region
[Use Π = 22/7]

Calculate the area of the designed region in the given figure common between the two quadrants of circles of radius 8 cm each. [Use Π = 22/7]

The area enclosed between the concentric circles is 770cm2. If the radius of outer circle 21cm. find the radius of inner circle
If the area of an equilateral triangle is `36sqrt3 cm^2` find its perimeter.
Find the area of shaded region in Fig. 4, where a circle of radius 6 cm has been drawn with vertex O of an equilateral triangle OAB of side 12 cm. (Use π = 3.14 and\[\sqrt{3}\] =1.73)
Read the following passage:
Governing council of a local public development authority of Dehradun decided to build an adventurous playground on the top of a hill, which will have adequate space for parking.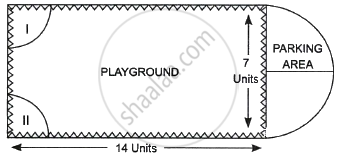 After survey, it was decided to build rectangular playground, with a semi-circular area allotted for parking at one end of the playground. The length and breadth of the rectangular playground are 14 units and 7 units, respectively. There are two quadrants of radius 2 units on one side for special seats. |
Based on the above information, answer the following questions:
- What is the total perimeter of the parking area?
- (a) What is the total area of parking and the two quadrants?
OR
(b) What is the ratio of area of playground to the area of parking area? - Find the cost of fencing the playground and parking area at the rate of ₹ 2 per unit.
In the given figure, AB and CD are diameters of a circle with centre O perpendicular to each other. If OA = 7 cm, find the area of shaded region.