Advertisements
Advertisements
Question
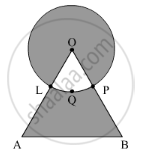
The area of an equilateral triangle ABC is 17320.5 cm2. With each vertex of the triangle as centre, a circle is drawn with radius equal to half the length of the side of the triangle (See the given figure). Find the area of shaded region. [Use π = 3.14 and `sqrt3 `= 1.73205]

Solution
Let the side of the equilateral triangle be a.
Area of equilateral triangle = 17320.5 cm2
sqrt3/4(s)^2 = 17320.5
1.7320/4a^2 = 17320.5
a2 = 4 x 10000
a = 200 cm
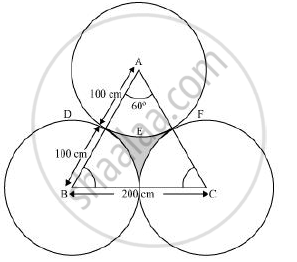
Each sector is of measure 60°.
Area of sector ADEF = `60^@/360^@ xx pixxr^2`
`=1/6xxpixx(100)^2`
`=(3.14xx10000)/6`
`= 15700/3 cm^2`
Area of shaded region = Area of equilateral triangle − 3 × Area of each sector
`= 17320.5 - 3xx 15700/3`
= 17320.5-15700 = 1620.5 cm2
APPEARS IN
RELATED QUESTIONS
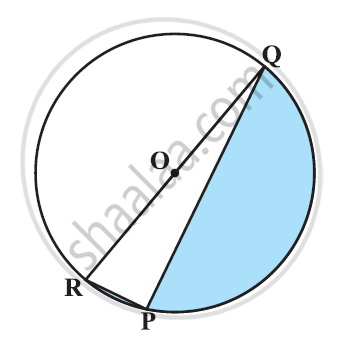
Find the area of the shaded region in the given figure, if PQ = 24 cm, PR = 7 cm and O is the centre of the circle. [Use Π = 22/7]
From each corner of a square of side 4 cm a quadrant of a circle of radius 1 cm is cut and also a circle of diameter 2 cm is cut as shown in the given figure. Find the area of the remaining portion of the square [Use Π = 22/7]

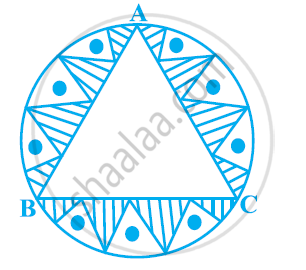
In a circular table cover of radius 32 cm, a design is formed leaving an equilateral triangle ABC in the middle as shown in the given figure. Find the area of the design (Shaded region). [Use Π = 22/7]
On a square handkerchief, nine circular designs each of radius 7 cm are made (see the given figure). Find the area of the remaining portion of the handkerchief.[Use Π = 22/7]

Find the area of triangle whose base measures 24 cm and the corresponding height measure 14.5 cm.
The perimeter of a triangular field is 240m, and its sides are in the ratio 25:17:12. Find the area of the field. Also, find the cost of ploughing the field at ₹ 40 per `m^2`
The difference between the sides at the right angles in a right-angled triangle is 7 cm. the area of the triangle is `60 cm^2` . Find its perimeter.
Find the area of shaded region in Fig. 4, where a circle of radius 6 cm has been drawn with vertex O of an equilateral triangle OAB of side 12 cm. (Use π = 3.14 and\[\sqrt{3}\] =1.73)
A plane left 30 minutes later than the scheduled time and in order to reach its destination 1500 km away on time, it has to increase its speed by 250 km/hr from its usual speed. Find the usual speed of the plane.
Find the area of the shaded region in figure.