Advertisements
Advertisements
Question
In the given figure PQ is a tangent to the circle at A, AB and AD are bisectors of `angleCAQ` and `angle PAC`. if `angleBAQ = 30^@. prove that:
1) BD is a diameter of the circle
2) ABC is an isosceles triangle

Solution
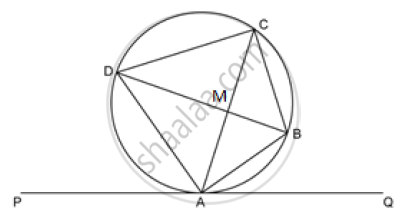
1) `angleBAQ = 30^@`
SinceAB is the bisector of `angleCAQ`
`=> angleCAB = angleBAQ = 30^@`
AD is the bisector of `angle CAP` and P-A-Q,
`angle DAP + angle CAD + angle CAQ = 180^@`
`=> angleCAD + anglle CAD + 60^@ = 180 ^@`
`=> angle CAD = 60^@`
So `angle CAD + angle CAB = 60^@ + 30^@ = 90^@`
Since angle in a semi-circle = 90°
⇒ Angle made by diameter to any point on the circle is 90°
So, BD is the diameter of the circle.
2) SinceBD is the diameter of the circle, so it will pass through the centre.
By Alternate segment theorem
`angle ABD = angle DAC = 60^@`
So, in `angle BMA`,
`angle AMB = 90^@` .........(UseAngleSumProperty)
We know that perpendicular drawn from the centre to a chord of a circle bisects the chord.
`=> angle BMA = angle BMC = 90^@`
In `triangleBMA` and `triangleBMC`
`angleBMA = angleBMC = 90^@`
BM = BM (common side)
AM = CM(perpendicular drawn from the centre to a chord of a circle bisects the chord.)
⇒ ΔBMA ≅ ΔBMC
⇒ AB = BC (SAS congruence criterion)
⇒ ΔABC is an isosceles triangle.
APPEARS IN
RELATED QUESTIONS
Draw a line segment AB of length 8 cm. Taking A as centre, draw a circle of radius 4 cm and taking B as centre, draw another circle of radius 3 cm. Construct tangents to each circle from the centre of the other circle.
In the figure given below, O is the centre of the circle and SP is a tangent. If ∠SRT = 65°,
find the value of x, y and z.

Construct a triangle ABC in which base BC = 5.5 cm, AB = 6 cm and ∠ABC = 120°.
- Construct a circle circumscribing the triangle ABC.
- Draw a cyclic quadrilateral ABCD so that D is equidistant from B and C.
Draw a circle of radius 3.5 cm. Take two points A and B on one of its extended diameter, each at a distance of 5 cm from its center. Draw tangents to the circle from each of these points A and B.
Construct a tangent to a circle of radius 4 cm form a point on the concentric circle of radius 6 cm and measure its length. Also, verify the measurement by actual calculation.
Draw a pair of tangents to a circle of radius 3 cm, which are inclined to each other at an angle of 60°.
Draw two concentric circles with radii 4 cm and 6 cm. Taking a point on the outer circle, construct a pair of tangents to inner circle. By measuring the lengths of both the tangents, show that they are equal to each other.
Draw a circle at a radius of 4 cm. Take a point on it. Without using the centre at the circle, draw a tangent to the circle at point P.
To draw a pair of tangents to a circle which are inclined to each other at an angle of 60°, it is required to draw tangents at end points of those two radii of the circle, the angle between them should be ______.
Using ruler and compass construct a triangle ABC in which AB = 6 cm, ∠BAC = 120° and AC = 5 cm. Construct a circle passing through A, B and C. Measure and write down the radius of the circle.
