Advertisements
Advertisements
Question
In how many points two distinct planes can intersect?
Solution
Two distinct planes can intersect at only one unique line, which is common to both of them and one line is made up of infinite points. Therefore, two distinct planes can intersect at infinite points.
APPEARS IN
RELATED QUESTIONS
The following statement is true or false? Give reason for your answer.
Only one line can pass through a single point.
Why is Axiom 5, in the list of Euclid’s axioms, considered a ‘universal truth’? (Note that the question is not about the fifth postulate.)
How many planes can be made to pass through a line and a point not on the line?
How many planes can be made to pass through three distinct points?
Euclid divided his famous treatise “The Elements” into ______.
Solve the following question using appropriate Euclid’s axiom:
It is known that x + y = 10 and that x = z. Show that z + y = 10?
Solve the following question using appropriate Euclid’s axiom:
In the following figure, we have ∠1 = ∠2, ∠2 = ∠3. Show that ∠1 = ∠3.
Solve the following question using appropriate Euclid’s axiom:
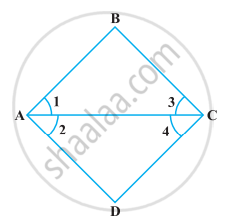
In the following figure, we have ∠ABC = ∠ACB, ∠3 = ∠4. Show that ∠1 = ∠2.

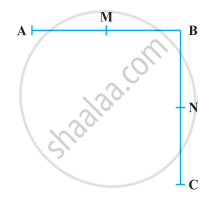
In the following figure AB = BC, M is the mid-point of AB and N is the mid-point of BC. Show that AM = NC.
Read the following statement :
An equilateral triangle is a polygon made up of three line segments out of which two line segments are equal to the third one and all its angles are 60° each.
Define the terms used in this definition which you feel necessary. Are there any undefined terms in this? Can you justify that all sides and all angles are equal in a equilateral triangle.
