Advertisements
Advertisements
Question
In the following figure AB = BC, M is the mid-point of AB and N is the mid-point of BC. Show that AM = NC.
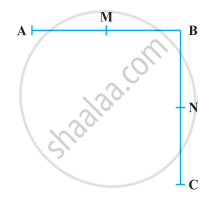
Solution
Given, AB = BC ...(i)
M is the mid-point of AB.
∴ AM = MB = `1/2` AB ...(ii)
And N is the mid-point of BC.
∴ BN = NC = `1/2` BC ...(iii)
According to Euclid’s axiom, things which are halves of the same things are equal to one another.
From equation (i), AB = BC
On multiplying both sides by `1/2`, we get
`1/2` AB = `1/2` BC
⇒ AM = NC ...[Using equations (ii) and (iii)]
APPEARS IN
RELATED QUESTIONS
Give a definition of the following term. Are there other terms that need to be defined first? What are they, and how might you define them?
perpendicular lines
Give a definition of the following term. Are there other terms that need to be defined first? What are they, and how might you define them?
square
How many lines can be drawn through both of the given points?
The number of dimensions, a solid has ______.
Euclid divided his famous treatise “The Elements” into ______.
In Indus Valley Civilisation (about 3000 B.C.), the bricks used for construction work were having dimensions in the ratio ______.
The side faces of a pyramid are ______.
Which of the following needs a proof?
“For every line l and for every point P not lying on a given line l, there exists a unique line m passing through P and parallel to l ” is known as Playfair’s axiom.
The following statement is true or false? Give reason for your answer.
If two circles are equal, then their radii are equal.
