Advertisements
Advertisements
Question
In the given figure, triangle ABC is a right-angled at B. D is the mid-point of side BC. Prove that AC2 = 4AD2 – 3AB2.

Solution
Given: ∠ABC = 90°, D is mid-point of side BC.
To prove: AC2 = 4AD2 – 3AB2.
Proof: Since D is mid-point of side BC,
∴ BD = DC = `1/2` BC ......(i)
Now, in ΔABC, by Pythagoras theorem,
AB2 + BC2 = AC2
⇒ AB2 + (2BD)2 = AC2 .....[Using (i)]
⇒ AB2 + 4BD2 = AC2 ......(iii)
Similarly, in ΔABD, by Pythagoras theorem,
AB2 + BD2 = AD2
⇒ BD2 = AD2 – AB2
Putting the value of BD2 in equation (ii),
AB2 + 4(AD2 – AB2) = AC2
⇒ AB2 + 4AD2 – 4AB2 = AC2
⇒ 4AD2 – 3AB2 = AC2
or AC2 = 4AD2 – 3AB2
Hence proved.
APPEARS IN
RELATED QUESTIONS
Adjacent sides of a parallelogram are 11 cm and 17 cm. If the length of one of its diagonal is 26 cm, find the length of the other.
In the given figure, seg PS is the median of ∆PQR and PT ⊥ QR. Prove that,
PR2 = PS2 + QR × ST + `("QR"/2)^2`

Height and base of a right angled triangle are 24 cm and 18 cm find the length of its hypotenuse
Some question and their alternative answer are given. Select the correct alternative.
In ∆ABC, AB = \[6\sqrt{3}\] cm, AC = 12 cm, BC = 6 cm. Find measure of ∠A.
Find the length a diagonal of a rectangle having sides 11 cm and 60 cm.
In ∆RST, ∠S = 90°, ∠T = 30°, RT = 12 cm then find RS and ST.
Prove that the sum of the squares of the diagonals of a parallelogram is equal to the sum of the squares of its sides.
Seg PM is a median of ∆PQR. If PQ = 40, PR = 42 and PM = 29, find QR.
Seg AM is a median of ∆ABC. If AB = 22, AC = 34, BC = 24, find AM
If hypotenuse of a right angled triangle is 5 cm, find the radius of
the circle passing through all vertices of the triangle.
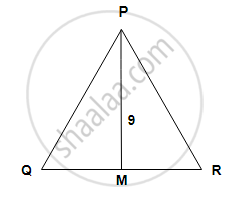
In ΔPQR, seg PM is a median, PM = 9 and PQ2 + PR2 = 290. Find the length of QR.
In ΔPQR, seg PM is the median. If PM = 9, PQ2 + PR2 = 290, Find QR.
In ΔABC, seg AP is a median. If BC = 18, AB2 + AC2 = 260, then find the length of AP.
Choose the correct alternative:
Out of given triplets, which is not a Pythagoras triplet?
Choose the correct alternative:
Out of all numbers from given dates, which is a Pythagoras triplet?
"The diagonals bisect each other at right angles." In which of the following quadrilaterals is the given property observed?
Which of the following figure is formed by joining the mid-points of the adjacent sides of a square?
