Advertisements
Advertisements
Question
Adjacent sides of a parallelogram are 11 cm and 17 cm. If the length of one of its diagonal is 26 cm, find the length of the other.
Solution
Let □PQRS be a parallelogram.
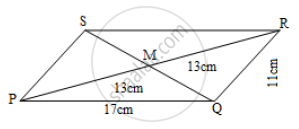
Then, PQ = 17 cm, QR = 11 cm and diagonal PR = 26 cm The diagonals of a parallelogram bisect each other. Point M is the point of intersection of diagonals PR and QS.
`therefore PM=MR=1/2PR=1/2xx26`
`therefore PM=MR=13 cm ......(1)`
`QM=1/2QS`
`thereforeQS=2QM......(2)`
In ΔPQR, QM is the median.
`PQ^2+QR^2=2PM^2+2QM^2` .......(By Apollonius theorem)
`(17)^2+(11)^2=2(13)^2+2QM^2`
`therefore 289+121=2(169)+2QM^2`
`therefore 410=2(169)+2QM^2`
Diving by 2, we get
`205 =169 + QM^2`
`therefore QM^2= 205 -169=36`
`therefore QM=6`
`therefore QS=2QM=2xx6=12cm`
Thus, the length of the other diagonal is 12 cm.
APPEARS IN
RELATED QUESTIONS
In ∆ABC, point M is the midpoint of side BC. If, AB2 + AC2 = 290 cm2, AM = 8 cm, find BC.

Some question and their alternative answer are given. Select the correct alternative.
Altitude on the hypotenuse of a right angled triangle divides it in two parts of lengths 4 cm and 9 cm. Find the length of the altitude.
Height and base of a right angled triangle are 24 cm and 18 cm find the length of its hypotenuse
Some question and their alternative answer are given. Select the correct alternative.
In ∆ABC, AB = \[6\sqrt{3}\] cm, AC = 12 cm, BC = 6 cm. Find measure of ∠A.
Do sides 7 cm, 24 cm, 25 cm form a right angled triangle ? Give reason
Find the length a diagonal of a rectangle having sides 11 cm and 60 cm.
In ∆RST, ∠S = 90°, ∠T = 30°, RT = 12 cm then find RS and ST.
Prove that the sum of the squares of the diagonals of a parallelogram is equal to the sum of the squares of its sides.
Sum of the squares of adjacent sides of a parallelogram is 130 sq.cm and length of one of its diagonals is 14 cm. Find the length of the other diagonal.
Choose the correct alternative:
Out of the following which is a Pythagorean triplet?
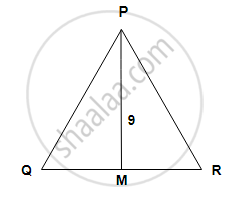
In ΔPQR, seg PM is the median. If PM = 9, PQ2 + PR2 = 290, Find QR.
In ΔABC, seg AP is a median. If BC = 18, AB2 + AC2 = 260, then find the length of AP.
Choose the correct alternative:
Out of given triplets, which is not a Pythagoras triplet?
Which of the following figure is formed by joining the mid-points of the adjacent sides of a square?
In the given figure, triangle ABC is a right-angled at B. D is the mid-point of side BC. Prove that AC2 = 4AD2 – 3AB2.

