Advertisements
Advertisements
Question
In a triangle ABC, line l || Side BC and line l intersects side AB and AC in points P and Q, respectively. Prove that: `"AP"/"BP"="AQ"/"QC"`

Solution
Construction: Join seg PC and seg BQ.

Given: In ΔABC , line l || BC
Line l intersects side AB and side AC in points P and Q, respectively.
A-P-B and A-Q-C
`"To Prove: ""AP"/"BP"="AQ"/"QC"`
Construction: Join seg BQ and seg CP
In ΔAPQ and ΔBPQ ,
`(A(triangleAPQ))/(A(triangleBPQ))=(AP)/(BP) " (i) ...........""(Triangles having equal height)"`
In ΔAPQ and ΔCPQ
`(A(triangleAPQ))/(A(triangleCPQ))=(AQ)/(CQ)" (ii) ......(Triangles having equal height)"`
`A(triangleBPQ)=A(triangleCPQ)" (iii) .....(Triangles with common base PQ and same height)"`
`(A(triangleAPQ))/(A(triangleBPQ))=(A(triangleAPQ))/(A(triangleCPQ))" ..............From (i), (ii) and (iii)"`
`"AP"/"BP"="AQ"/"QC"`
APPEARS IN
RELATED QUESTIONS
In the following figure, if LM || CB and LN || CD, prove that `("AM")/("AB")=("AN")/("AD")`

Using Basic proportionality theorem, prove that a line drawn through the mid-points of one side of a triangle parallel to another side bisects the third side. (Recall that you have proved it in Class IX).
Using Converse of basic proportionality theorem, prove that the line joining the mid-points of any two sides of a triangle is parallel to the third side. (Recall that you have done it in Class IX).
In the figure, given below, the medians BD and CE of a triangle ABC meet at G. Prove that:

- ΔEGD ~ ΔCGB and
- BG = 2GD from (i) above.
The two similar triangles are equal in area. Prove that the triangles are congruent.
In a circle, two chords AB and CD intersect at a point P inside the circle. Prove that
(a) ΔPAC ∼PDB (b) PA. PB= PC.PD

In the given figure, ∠PQR = ∠PST = 90° ,PQ = 5cm and PS = 2cm.
(i) Prove that ΔPQR ~ ΔPST.
(ii) Find Area of ΔPQR : Area of quadrilateral SRQT.

If Δ ABC , MN || BC .

If AN : AC= 5 : 8, find ar(Δ AMN) : ar(Δ ABC)
In Δ ABC, DE || BC; DC and EB intersects at F. if `"DE"/"BC" = 2/7` , find `("Ar" (triangle "FDE"))/("Ar" (triangle "FBC"))`
The scale of a map is 1 : 200000. A plot of land of area 20km2 is to be represented on the map. Find:
The area in km2 that can be represented by 1 cm2
A triangle ABC is enlarged, about the point O as centre of enlargement, and the scale factor is 3. Find : OC', if OC = 21 cm.
Also, state the value of :
- `(OB^')/(OB)`
- `(C^'A^')/(CA)`
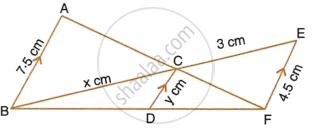
In the figure, given below, AB, CD and EF are parallel lines. Given AB = 7.5 cm, DC = y cm, EF = 4.5 cm, BC = x cm and CE = 3 cm, calculate the values of x and y.
Sides of a triangle are 7, 24 and 25. Determine whether the triangle is a right-angled triangle or not.
The scale of a map is 1 : 50000. The area of a city is 40 sq km which is to be represented on the map. Find: The length of a scale in km represented by 1cm on the map.
A map is drawn to scale of 1:20000. Find: The area of the lake on the map which has an actual area of 12km2
If ∆ABC is an isosceles triangle with ∠C = 90° and AC = 5 cm, then AB is
PR = 26 cm, QR = 24 cm, ∠PAQ = 90°, PA = 6 cm and QA = 8 cm. Find ∠PQR
In any triangle _______ sides are opposite to equal angles
In fig. BP ⊥ AC, CQ ⊥ AB, A−P−C, and A−Q−B then show that ΔAPB and ΔAQC are similar.
In ΔAPB and ΔAQC
∠APB = [ ]° ......(i)
∠AQC = [ ]° ......(ii)
∠APB ≅ ∠AQC .....[From (i) and (ii)]
∠PAB ≅ ∠QAC .....[______]
ΔAPB ~ ΔAQC .....[______]
In figure, if PQRS is a parallelogram and AB || PS, then prove that OC || SR.
