Advertisements
Advertisements
Question
In two concentric circles, prove that all chords of the outer circle, which touch the inner circle, are of equal length.
Solution 1
i. PQ = RQ
∴ ∠PRQ = ∠QPR ...(Opposite angles of equal sides of a triangle)
`=>` ∠PRQ + ∠QPR + 68° = 180°
`=>` 2∠PRQ = 180° – 68°
`=> ∠PRQ = (112^circ)/2 = 56^circ`
Now, ∠QOP = 2∠PRQ ...(Angle at the centre is double)
`=>` QOP = 2 × 56° = 112°
ii. ∠PQC = ∠PRQ ...(Angles in alternate segments are equal)
∠QPC = ∠PRQ ...(Angles in alternate segments)
∴ ∠PQC = ∠QPC = 56° ...(∵ ∠PRQ = 56° from(i))
∠PQC + ∠QPC + ∠QCP = 180°
`=>` 56° + 56° + ∠QCP = 180°
`=>` ∠QCP = 68°
Solution 2
Consider two concentric circles with centres at O. Let AB and CD be two chords of the outer circle which touch the inner circle at the points M and N respectively.

To prove the given question, it is sufficient to prove AB = CD.
For this join OM, ON, OB and OD.
Let the radius of outer and inner circles be R and r respectively.
AB touches the inner circle at M.
AB is a tangent to the inner circle
∴ OM ⊥ AB
`=>` BM = `1/2` AB
`=>` AB = 2BM
Similarly ON ⊥ CD, and CD = 2DN
Using pythagoras theorem in ΔOMB and ΔOND
OB2 = OM2 + BM2, OD2 = ON2 + DM2
`=> "BM" = sqrt ("R"^2 - "r"^2), "DN" = sqrt ("R"^2 - "r"^2)`
Now,
AB = 2BM = `2 sqrt ("R"^2 - "r"^2) , "CD" = 2"DN" = 2 sqrt ("R"^2 - "r"^2)`
∴ AB = CD
Hence Proved.
APPEARS IN
RELATED QUESTIONS
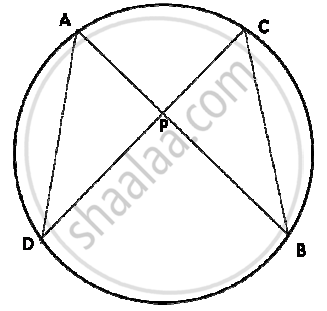
AB and CD are two chords of a circle intersecting at P. Prove that AP x PB = CP x PD
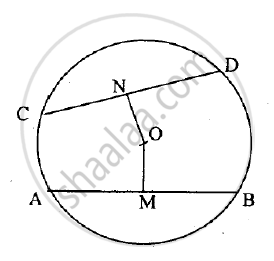
In the figure given below, O is the centre of the circle. AB and CD are two chords of the circle. OM is perpendicular to AB and ON is perpendicular to CD.
AB = 24 cm, OM= 5 cm, ON= 12 cm. Find the:
1) radius of the circle
2) length of chord CD.
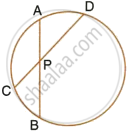
In the given figure 3 × CP = PD = 9 cm and AP = 4.5 cm. Find BP.
In the given figure, 5 × PA = 3 × AB = 30 cm and PC = 4 cm. Find CD.
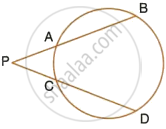
In the given figure, tangent PT = 12.5 cm and PA = 10 cm; find AB.
Prove that, of any two chords of a circle, the greater chord is nearer to the centre.
Chords AB and CD of a circle intersect each other at point P such that AP = CP
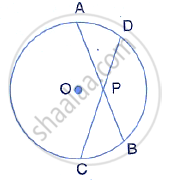
Show that: AB = CD
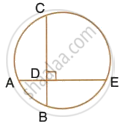
In the given figure, AE and BC intersect each other as point D. If ∠CDE = 90°, AB = 5 cm, BD = 4 cm and CD = 9 cm, find AE.
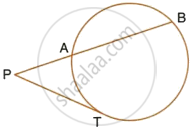
Two tangents are drawn to a circle from an external point P. touching the circle at the points A and B. A third tangent intersects segment PA in C and segment PB in D and touches the circle at Q. if PA = 20 units, find the perimeter of Δ PCD.
Two circles of radii 10 cm and 8 cm intersect and the length of the common chord is 12 cm. Find the distance between their centres.
