Advertisements
Advertisements
Question
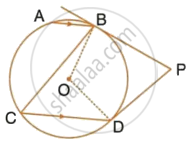
In the given figure, O is the centre of the circle. The tangents at B and D intersect each other at point P. If AB is parallel to CD and ∠ABC = 55°, find:
- ∠BOD
- ∠BPD
Solution
In the given figure, O is the centre of the circle AB || CD, ∠ABC = 55° tangents at B and D are drawn which meet at P.

∵ AB || CD
∴ ∠ABC = ∠BCD ...(Alternate angles)
∴ ∠ABC = 55° ...(Given)
i. Now arc BD subtands ∠BOD at the centre and ∠BCD at the remaining part of the circle.
∴ ∠BOD = 2∠BCD
= 2 × 55°
= 110°
ii. In quadrilateral OBPD,
∠OBP = ∠ODP = 90° ...(∵ BP and DP are tangents)
∴ ∠BOD + ∠BPD = 180°
`\implies` 110° + ∠BPD = 180°
`\implies` ∠BPD = 180° – 110° = 70°
Hence, ∠BOD = 110° and ∠BPD = 70°
APPEARS IN
RELATED QUESTIONS
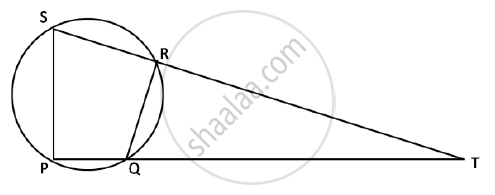
In the given figure PQRS is a cyclic quadrilateral PQ and SR produced meet at T
1) Prove ΔTPS ~ ΔTRQ.
2) Find SP if TP = 18 cm, RQ = 4 cm and TR = 6 cm
3) Find the area of quadrilateral PQRS if the area of ΔPTS = 27 cm2.
PQRS is a cyclic quadrilateral. Given ∠QPS = 73°, ∠PQS = 55° and ∠PSR = 82°, calculate:
1) ∠QRS
2) ∠RQS
3) ∠PRQ

In the given figure, AB is the diameter of a circle with centre O. ∠BCD = 130o. Find:
1) ∠DAB
2) ∠DBA

In the following figure,
- if ∠BAD = 96°, find ∠BCD and ∠BFE.
- Prove that AD is parallel to FE.

In the given figure, SP is bisector of ∠RPT and PQRS is a cyclic quadrilateral. Prove that : SQ = SR.

In the following figure, ABCD is a cyclic quadrilateral in which AD is parallel to BC.

If the bisector of angle A meets BC at point E and the given circle at point F, prove that:
- EF = FC
- BF = DF
In a cyclic quadrilateral ABCD , AB || CD and ∠ B = 65 ° , find the remaining angles

In following fig., O is the centre of the circle. Find ∠ CBD.

ABCD is a cyclic quadrilateral AB and DC are produced to meet in E. Prove that Δ EBC ∼ Δ EDA.
In the given circle with centre O, ∠ABC = 100°, ∠ACD = 40° and CT is a tangent to the circle at C. Find ∠ADC and ∠DCT.

