Advertisements
Advertisements
Question
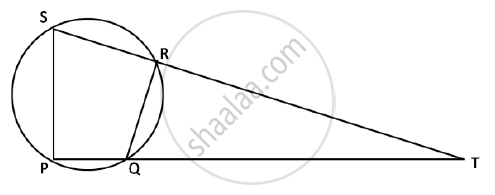
In the given figure PQRS is a cyclic quadrilateral PQ and SR produced meet at T
1) Prove ΔTPS ~ ΔTRQ.
2) Find SP if TP = 18 cm, RQ = 4 cm and TR = 6 cm
3) Find the area of quadrilateral PQRS if the area of ΔPTS = 27 cm2.
Solution
1) Since `square PQRS` is a cyclic quadrilateral
`angle QRT = angle SPT` ....(1)(exterior angle is equal to interior opposite angle)
In ΔTPS and ΔTRQ
`angle PTS = angle RTQ` . . . .(common angle)
`angleQRT = angle SPT` . . . .(from 1)
⇒ ΔTPS ~ ΔTRQ . . . .(AA similarity criterion)
2) Since ΔTPS ~ ΔTRQ, implies that corresponding sides are proportional
`i.e (SP)/(QR) = (TP)/(TR)`
`=> (SP)/4 = 18/6`
`=> (SP)= (18xx4)/6`
`=> SP = 12cm`
3) Since ΔTPS ~ ΔTRQ
`(Ar(ΔTPS))/(Ar(ΔTRQ)) = "SP"^2/"RQ"^2`
`=> 27/(Ar(ΔTRQ)) = (27xx4xx4)/(12xx12)`
`=> Ar(ΔTRQ) = 3cm^2`
Now, Ar(`square PQRS`) = Ar(ΔTPS) Ar(ΔTRQ)= 27 - 3 = 24 cm2
APPEARS IN
RELATED QUESTIONS
In the given figure, SP is bisector of ∠RPT and PQRS is a cyclic quadrilateral. Prove that : SQ = SR.

In the given figure, PAT is tangent to the circle with centre O at point A on its circumference and is parallel to chord BC. If CDQ is a line segment, show that:
- ∠BAP = ∠ADQ
- ∠AOB = 2∠ADQ
- ∠ADQ = ∠ADB
In a cyclic quadrilateral ABCD , AB || CD and ∠ B = 65 ° , find the remaining angles

In triangle ABC, AB = AC. A circle passing through B and c intersects the sides AB and AC at D and E respectively. Prove that DE || BC.
Bisectors of angles A, B and C of a triangle ABC intersect its circumcircle at D, E and F respectively. Prove that the angles of Δ DEF are 90° - `"A"/2` , 90° - `"B"/2` and 90° - `"C"/2` respectively.
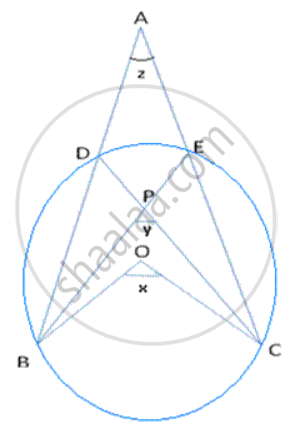
In following fig., O is the centre of the circle, prove that ∠x =∠ y + ∠ z.
In the figure, O is the centre of the circle and the length of arc AB is twice the length of arc BC. If angle AOB = 108°, find: ∠ADB.
In the figure, ∠DBC = 58°. BD is a diameter of the circle. Calculate : ∠BAC

Prove that the angle bisectors of the angles formed by producing opposite sides of a cyclic quadrilateral (Provided they are not parallel) intersect at the right angle.
In Fig. ABCD is a cyclic quadrilateral. A circle passing through A and B meets AD and BC in the points E and F respectively. Prove that EF || DC.