Question

Bisectors of angles A, B and C of a triangle ABC intersect its circumcircle at D, E and F respectively. Prove that the angles of  Δ DEF are 90° - `"A"/2` , 90° - `"B"/2` and 90° - `"C"/2` respectively.

Answer 1

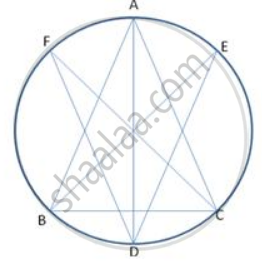
Since AD , BE and CF are bisectors of ∠ A , ∠ B and ∠ C respectively.

∴ ∠1 = ∠ 2 = ∠`"A"/2`

∠3 = ∠4 = ∠`"B"/2`

∠5= ∠6 = ∠`"C"/2`

∠ADE = ∠3  ....(1)

Also ∠ADF = ∠6  ....(2)  (angles in the same segment)

Adding (1) and (2)

∠ADE + ∠ADF = ∠3 + ∠6

∠D = `1/2`∠B + `1/2` ∠C

∠D = `1/2` (B + ∠C) = `1/2` (180 - ∠A) (∠A + ∠B + ∠C = 180°)

∠D = 90 - `1/2` ∠A

Similarly ,

∠E = 90 - `1/2` ∠B , ∠F = 90 - `1/2` ∠C