Selina solutions for Mathematics [English] Class 10 ICSE chapter 18 - Tangents and Intersecting Chords [Latest edition]

The radius of a circle is 8 cm. calculate the length of a tangent draw to this circle from a point at a distance of 10 cm from its centre.

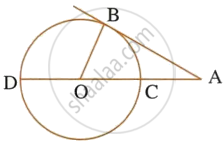
In the given figure, O is the center of the circle and AB is a tangent at B. If AB = 15 cm and AC = 7.5 cm, calculate the radius of the circle.

Two circle touch each other externally at point P. Q is a point on the common tangent through P. Prove that the tangents QA and QB are equal.

Two circle touch each other internally. Show that the tangents drawn to the two circles from any point on the common tangent are equal in length.

Two circles of radii 5 cm and 3 cm are concentric. Calculate the length of a chord of the outer circle which touches the inner.

Three circles touch each other externally. A triangle is formed when the centres of these circles are joined together. Find the radii of the circle, if the sides of the triangle formed are 6 cm, 8 cm and 9 cm.

If the sides of a quadrilateral ABCD touch a circle, prove that : AB + CD = BC + AD.

If the sides of a parallelogram touch a circle in following figure, prove that the parallelogram is a rhombus.

From the given figure, prove that : AP + BQ + CR = BP + CQ + AR. 

Also show that : AP + BQ + CR = `1/2` × Perimeter of ΔABC.

In the following figure; If AB = AC then prove that BQ = CQ.

Radii of two circles are 6.3 cm and 3.6 cm. State the distance between their centres if:

1. they touch each other externally,
2. they touch each other internally.

Radii of two circles are 6.3 cm and 3.6 cm. State the distance between their centers if -

they touch each other internally.

From a point P outside a circle, with centre O, tangents PA and PB are drawn. Prove that:

∠AOP = ∠BOP

From a point P outside a circle, with centre O, tangents PA and PB are drawn. Prove that:

OP is the ⊥ bisector of chord AB.

In the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that :

1. tangent at point P bisects AB,
2. angles APB = 90°.

In the given figure, two circles touch each other externally at point P. AB is the direct common tangent of these circles. Prove that: 

(ii) angles APB = 90°

Tangents AP and AQ are drawn to a circle, with centre O, from an exterior point A. Prove that : ∠PAQ = 2∠OPQ

Two parallel tangents of a circle meet a third tangent at points P and Q. Prove that PQ subtends a right angle at the centre.

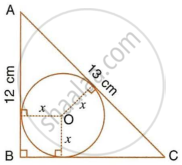
ABC is a right angles triangle with AB = 12 cm and AC = 13 cm. A circle, with centre O, has been inscribed inside the triangle.

Calculate the value of x, the radius of the inscribed circle.

In a triangle ABC, the incircle (centre O) touches BC, CA and AB at points P, Q and R respectively. Calculate : 

1. ∠QOR
2. ∠QPR;

given that ∠A = 60°.

In a triangle ABC, the incircle (centre O) touches BC, CA and AB at points P, Q and R respectively. Calculate:

i)`∠`QPR .

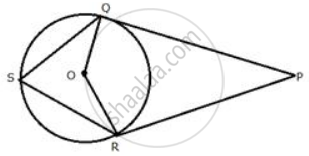
In the following figure, PQ and PR are tangents to the circle, with centre O. If `∠`QPR = 60°, calculate:

1. ∠QOR,
2. ∠OQR,
3. ∠QSR.

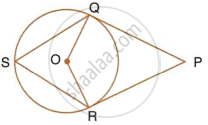
In the following figure, PQ and PR are tangents to the circle, with centre O. If ∠ QPR = 60° , calculate:

∠ OQR 

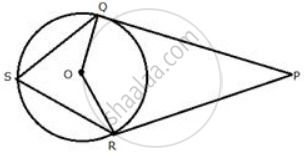
In the following figure, PQ and PR are tangents to the circle, with centre O. If ∠ QPR = 60° , calculate:

∠ QSR 

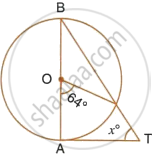
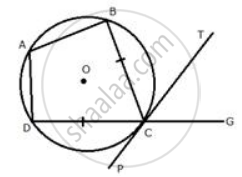
In the given figure, AB is the diameter of the circle, with centre O, and AT is the tangent. Calculate the numerical value of x.

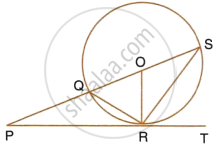
In quadrilateral ABCD; angles D = 90°, BC = 38 cm and DC = 25 cm. A circle is inscribed in this quadrilateral which touches AB at point Q such that QB = 27 cm, Find the radius of the circle.

In the given figure, PT touches the circle with centre O at point R. Diameter SQ is produced to meet the tangent TR at P. Given ∠SPR = x° and ∠QRP = y°;

Prove that:

1. ∠ORS = y°
2. write an expression connecting x and y.

PT is a tangent to the circle at T. If ∠ABC = 70° and ∠ACB = 50°; calculate:

1. ∠CBT
2. ∠BAT
3. ∠APT

PT is a tangent to the circle at T. If ∠ ABC = 70° and ∠ ACB = 50° ; calculate : ∠ APT

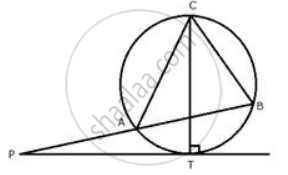
PT is a tangent to the circle at T. If ∠ ABC = 70° and ∠ ACB = 50° ; calculate : ∠ APT

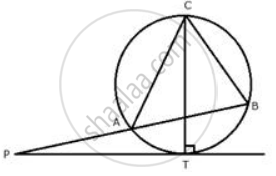
In the given figure, O is the centre of the circumcircle ABC. Tangents at A and C intersect at P. Given angle AOB = 140° and angle APC = 80°; find the angle BAC.

In the given figure, PQ is a tangent to the circle at A. AB and AD are bisectors of ∠CAQ and ∠PAC. If ∠BAQ = 30°, prove that : BD is diameter of the circle.

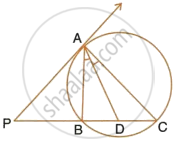
In the given figure 3 × CP = PD = 9 cm and AP = 4.5 cm. Find BP. 

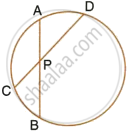
In the given figure, 5 × PA = 3 × AB = 30 cm and PC = 4 cm. Find CD.

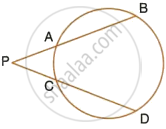
In the given figure, tangent PT = 12.5 cm and PA = 10 cm; find AB.

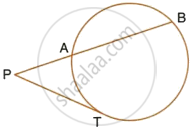
In the given figure, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find AB.

In the given figure, diameter AB and chord CD of a circle meet at P. PT is a tangent to the circle at T. CD = 7.8 cm, PD = 5 cm, PB = 4 cm. Find the length of tangent PT.

In the following figure, PQ is the tangent to the circle at A, DB is the diameter and O is the centre of the circle. If ∠ADB = 30° and ∠CBD = 60°, calculate:

1. ∠QAB, 
2. ∠PAD, 
3. ∠CDB.

In the following figure, PQ is the tangent to the circle at A, DB is a diameter and O is the centre of the circle. If ∠ ADB = 30° and ∠ CBD = 60° ; calculate ∠ PAD.

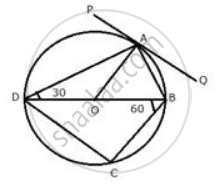
In the following figure, PQ is the tangent to the circle at A, DB is a diameter and O is the centre of the circle. If ∠ ADB = 30° and ∠ CBD = 60° ; calculate : ∠CDB

If PQ is a tangent to the circle at R; calculate:

1. ∠PRS,
2. ∠ROT.

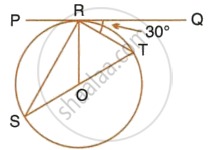
Given O is the centre of the circle and angle TRQ = 30°.

AB is the diameter and AC is a chord of a circle with centre O such that angle BAC = 30°. The tangent to the circle at C intersects AB produced in D. show that BC = BD.

Tangent at P to the circumcircle of triangle PQR is drawn. If the tangent is parallel to side, QR show that ΔPQR is isosceles.

Two circle with centres O and O' are drawn to intersect each other at points A and B. Centre O of one circle lies on the circumference of the other circle and CD is drawn tangent to the circle with centre O' at A. Prove that OA bisects angle BAC.

Two circles touch each other internally at a point P. A chord AB of the bigger circle intersects the other circle in C and D. Prove that ∠CPA = ∠DPB.

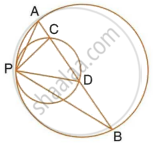
In a cyclic quadrilateral ABCD, the diagonal AC bisects the angle BCD. Prove that the diagonal BD is parallel to the tangent to the circle at point A.

In the figure, ABCD is a cyclic quadrilateral with BC = CD. TC is tangent to the circle at point C and DC is produced to point G. If ∠BCG = 108° and O is the centre of the circle, find :

1. angle BCT
2. angle DOC

In the figure, ABCD is a cyclic quadrilateral with BC = CD. TC is tangent to the circle at point C and DC is produced to point G. If angle BCG=108°  and O is the centre of the circle, find: angle DOC

Two circles intersect each other at points A and B. A straight line PAQ cuts the circles at P and Q. If the tangents at P and Q intersect at point T; show that the points P, B, Q and T are concyclic.

In the figure; PA is a tangent to the circle, PBC is secant and AD bisects angle BAC. Show that triangle PAD is an isosceles triangle. Also, show that:

`∠CAD = 1/2 (∠PBA - ∠PAB)`

Two circles intersect each other at points A and B. Their common tangent touches the circles at points P and Q as shown in the figure. Show that the angles PAQ and PBQ are supplementary.

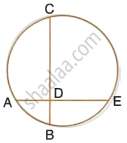
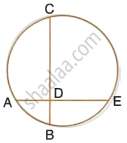
In the figure, chords AE and BC intersect each other at point D. If ∠CDE = 90°, AB = 5 cm, BD = 4 cm and CD = 9 cm; find DE.

In the figure, chords AE and BC intersect each other at point D. If AD = BD, show that AE = BC.

Circles with centres P and Q intersect at points A and B as shown in the figure. CBD is a line segment and EBM is tangent to the circle, with centre Q, at point B. If the circle are congruent; show that CE = BD.

In the adjoining figure, O is the centre of the circle and AB is a tangent to it at point B. ∠BDC = 65°. Find ∠BAO.

Prove that, of any two chords of a circle, the greater chord is nearer to the centre.

OABC is a rhombus whose three vertices A, B and C lie on a circle with centre O. If the radius of the circle is 10 cm, find the area of the rhombus.

OABC is a rhombus whose three vertices A, B and C lie on a circle with centre O. If the area of the rhombus is `32sqrt(3)  cm^2` find the radius of the circle.

Two circle with centres A and B, and radii 5 cm and 3 cm, touch each other internally. If the perpendicular bisector of the segment AB meets the bigger circle in P and Q; find the length of PQ.

Two chords AB and AC of a circle are equal. Prove that the centre of the circle lies on the bisector of angle BAC.

The diameter and a chord of a circle have a common end-point. If the length of the diameter is 20 cm and the length of the chord is 12 cm, how far is the chord from the centre of the circle?

ABCD is a cyclic quadrilateral in which BC is parallel to AD, angle ADC = 110° and angle BAC = 50°. Find angle DAC and angle DCA.

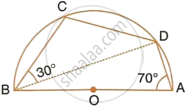
In the given figure, C and D are points on the semi-circle described on AB as diameter. Given angle BAD = 70° and angle DBC = 30°, calculate angle BDC.

In cyclic quadrilateral ABCD, ∠A = 3∠C and ∠D = 5∠B. Find the measure of each angle of the quadrilateral.

Show that the circle drawn on any one of the equal sides of an isosceles triangle as diameter bisects the base.

Bisectors of vertex angles A, B, and C of a triangle ABC intersect its circumcircle at the points D, E and F respectively. Prove that angle EDF  = 90° – `1/2` ∠A.

In the figure, AB is the chord of a circle with centre O and DOC is a line segment such that BC = DO. If ∠C = 20°, find angle AOD.

Prove that the perimeter of a right triangle is equal to the sum of the diameter of its incircle and twice the diameter of its circumcircle.

P is the mid-point of an arc APB of a circle. Prove that the tangent drawn at P will be parallel to the chord AB.

In the given figure, MN is the common chord of two intersecting circles and AB is their common tangent.

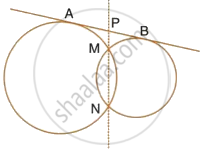
Prove that the line NM produced bisects AB at P.

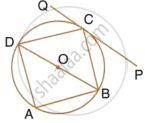
In the given figure, ABCD is a cyclic quadrilateral, PQ is tangent to the circle at point C and BD is its diameter. If ∠DCQ = 40° and ∠ABD = 60°, find;

1. ∠DBC
2. ∠BCP
3. ∠ADB

The given figure shows a circle with centre O and BCD is tangent to it at C. Show that : ∠ACD + ∠BAC = 90°.

ABC is a right triangle with angle B = 90°, A circle with BC as diameter meets hypotenuse AC at point D. Prove that: AC × AD = AB²

ABC is a right triangle with angle B = 90º. A circle with BC as diameter meets by hypotenuse AC at point D. Prove that: BD² = AD × DC.

In the given figure, AC = AE. Show that:

1. CP = EP
2. BP = DP

ABCDE is a cyclic pentagon with centre of its circumcircle at point O such that AB = BC = CD and angle ABC = 120°.

Calculate:

1. ∠BEC
2. ∠BED 

ABCDE is a cyclic pentagon with centre of its circumcircle at point O such that AB = BC = CD and angle ABC=120°.

Calculate: ∠ BED.

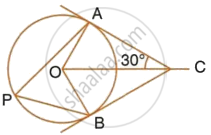
In the given figure, O is the centre of the circle. Tangents at A and B meet at C. If ∠ACO = 30°, find:

1. ∠BCO 
2. ∠AOB
3. ∠APB

In the given figure, O is the centre of the circle. Tangents at A and B meet at C. If angle ACO = 30°, find: angle AOB

In the given figure, O is the centre of the circle. Tangents at A and B meet at C. If angle ACO = 30°, find: angle APB

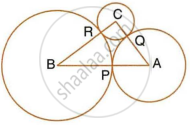
ABC is a triangle with AB = 10 cm, BC = 8 cm and AC = 6 cm (not drawn to scale). Three circle are drawn touching each other with the vertices as their centres. Find the radii of the three circles.

In a square ABCD, its diagonals AC and BD intersect each other at point O. The bisector of angle DAO meets BD at point M and the bisector of angle ABD meets AC at N and AM at L. Show that:

1. ∠ONL + ∠OML = 180°
2. ∠BAM + ∠BMA
3. ALOB is a cyclic quadrilateral.

In a square ABCD, its diagonal AC and BD intersect each other at point O. The bisector of angle DAO meets BD at point M and bisector of angle ABD meets AC at N and AM at L. Show that -  ∠ BAM = ∠ BMA

In a square ABCD, its diagonal AC and BD intersect each other at point O. The bisector of angle DAO meets BD at point M and bisector of angle ABD meets AC at N and AM at L. Show that - ALOB is a cyclic quadrilateral.

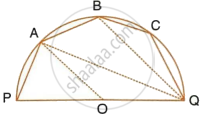
The given figure shows a semi-circle with centre O and diameter PQ. If PA = AB and ∠BCQ =140°; find measures of angles PAB and AQB. Also, show that AO is parallel to BQ.

The given figure shows a circle with centre O such that chord RS is parallel to chord QT, angle PRT = 20° and angle POQ = 100°. Calculate:

1. angle QTR
2. angle QRP
3. angle QRS
4. angle STR

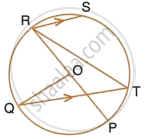
The given figure shows a circle with centre O such that chord RS is parallel to chord QT, angle PRT = 20° and angle POQ = 100°. Calculate:

(ii) angle QRP

The given figure shows a circle with centre O such that chord RS is parallel to chord QT, angle PRT = 20° and angle POQ = 100°. Calculate:

(iii) angle QRS

The given figure shows a circle with centre O such that chord RS is parallel to chord QT, angle PRT = 20° and angle POQ = 100°. Calculate: 

(iv) angle STR

In the given figure, PAT is tangent to the circle with centre O at point A on its circumference and is parallel to chord BC. If CDQ is a line segment, show that:

1. ∠BAP = ∠ADQ
2. ∠AOB = 2∠ADQ
3. ∠ADQ = ∠ADB

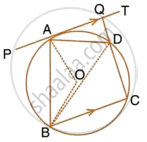
TA and TB are tangents to a circle with centre O from an external point T. OT intersects the circle at point P. Prove that AP bisects the angle TAB.

Two circles intersect in points P and Q. A secant passing through P intersects the circles in A and B respectively. Tangents to the circles at A and B intersect at T. Prove that A, Q, B and T lie on a circle.

Prove that any four vertices of a regular pentagon are concylic (lie on the same circle).

Chords AB and CD of a circle when extended meet at point X. Given AB = 4 cm, BX = 6 cm and XD = 5 cm, calculate the length of CD.

In the given figure, find TP if AT = 16 cm and AB = 12 cm.

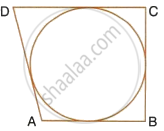
In the following figure, a circle is inscribed in the quadrilateral ABCD.

If BC = 38 cm, QB = 27 cm, DC = 25 cm and that AD is perpendicular to DC, find the radius of the circle.

In the given figure, XY is the diameter of the circle and PQ is a tangent to the circle at Y.

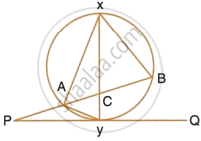
If ∠AXB = 50° and ∠ABX = 70°, find ∠BAY and ∠APY.

In the given figure, QAP is the tangent at point A and PBD is a straight line.

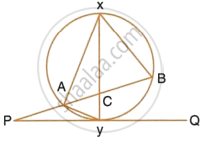
If ∠ACB = 36° and ∠APB = 42°, find:

1. ∠BAP
2. ∠ABD
3. ∠QAD
4. ∠BCD

In the given figure, AB is the diameter. The tangent at C meets AB produced at Q.
If ∠CAB = 34°, Find : ∠CBA

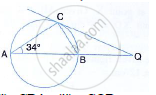
In the given figure, AB is the diameter. The tangent at C meets AB produced at Q.

If ∠ CAB = 34° , find : ∠ CQB

In the given figure, O is the centre of the circle. The tangents at B and D intersect each other at point P. If AB is parallel to CD and ∠ABC = 55°, find:

1. ∠BOD
2. ∠BPD

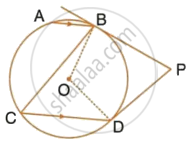
In two concentric circles, prove that all chords of the outer circle, which touch the inner circle, are of equal length.

In the following figure, PQ = QR, ∠RQP  = 68°, PC and CQ are tangents to the circle with centre O.

Calculate the values of:

1. ∠QOP
2. ∠QCP

In the figure, given below, AC is a transverse common tangent to two circles with centres P and Q and of radii 6 cm and 3 cm respectively.

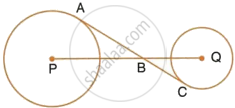
Given that AB = 8 cm, calculate PQ.

In the figure, given below, O is the centre of the circumcircle of triangle XYZ.

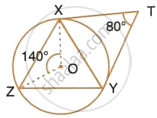
Tangents at X and Y intersect at point T. Given ∠XTY = 80° and ∠XOZ = 140°, calculate the value of ∠ZXY.

In the given figure, AE and BC intersect each other as point D. If ∠CDE = 90°, AB = 5 cm, BD = 4 cm and CD = 9 cm, find AE.

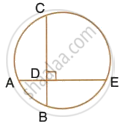
In the given circle with center o, ∠ABC=100°, ∠ACD=40° and CT is tangent to the circle at C. find ∠ADC and ∠DCT. 

In the figure given below, O is the center of the circle and SP is a tangent. If ∠SRT = 65°, find the value of x, y and Z.