Advertisements
Advertisements
Question
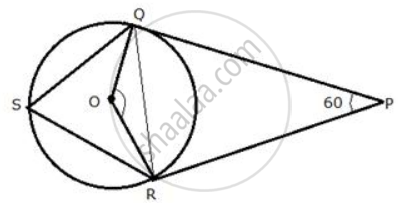
In the following figure, PQ and PR are tangents to the circle, with centre O. If `∠`QPR = 60°, calculate:
- ∠QOR,
- ∠OQR,
- ∠QSR.
Solution
Join QR.
i. In quadrilateral ORPQ,
OQ ⊥ OP, OR ⊥ RP
∴ ∠OQP = 90°, ∠ORP = 90°, ∠QPR = 60°
∠QOR = 360° – (90° + 90° + 60°)
∠QOR = 360° – 240°
∠QOR = 120°
ii. In ΔQOR,
OQ = QR ...(Radii of the same circle)
∴ ∠OQR = ∠QRO ...(i)
But, ∠OQR + ∠QRO + ∠QOR = 180°
∠OQR + ∠ QRO + 120° = 180°
∠OQR + ∠QRO = 60°
From (i)
2∠OQR = 60°
∠OQR = 30°
iii. Now arc RQ subtends ∠QOR at the centre and ∠QSR at the remaining part of the circle.
∴ `∠QSR = 1/2 ∠QOR`
`=> ∠QSR = 1/2 xx 120^circ`
`=>` ∠QSR = 60°
APPEARS IN
RELATED QUESTIONS
If the sides of a parallelogram touch a circle in following figure, prove that the parallelogram is a rhombus.
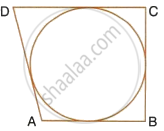
From the given figure, prove that : AP + BQ + CR = BP + CQ + AR.

Also show that : AP + BQ + CR = `1/2` × Perimeter of ΔABC.
If PQ is a tangent to the circle at R; calculate:
- ∠PRS,
- ∠ROT.
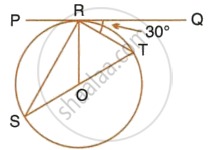
Given O is the centre of the circle and angle TRQ = 30°.
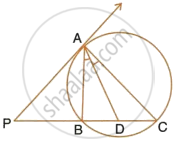
In the figure; PA is a tangent to the circle, PBC is secant and AD bisects angle BAC. Show that triangle PAD is an isosceles triangle. Also, show that:
`∠CAD = 1/2 (∠PBA - ∠PAB)`
In the given figure, AC = AE. Show that:
- CP = EP
- BP = DP

TA and TB are tangents to a circle with centre O from an external point T. OT intersects the circle at point P. Prove that AP bisects the angle TAB.
In the given figure, XY is the diameter of the circle and PQ is a tangent to the circle at Y.
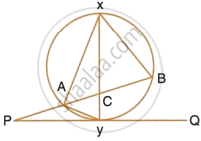
If ∠AXB = 50° and ∠ABX = 70°, find ∠BAY and ∠APY.
In the figure, PM is a tangent to the circle and PA = AM. Prove that:
(i) Δ PMB is isosceles
(ii) PA x PB = MB2

In Fig. AP is a tangent to the circle at P, ABC is secant and PD is the bisector of ∠BPC. Prove that ∠BPD = `1/2` (∠ABP - ∠APB).
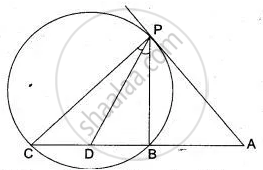
In the given figure PT is a tangent to the circle. Chord BA produced meets the tangent PT at P.
Given PT = 20 cm and PA = 16 cm.
- Prove ΔPTB ~ ΔPAT
- Find the length of AB.